Prove that a diagonal of a Parallelogram divides it into two congruent triangles.

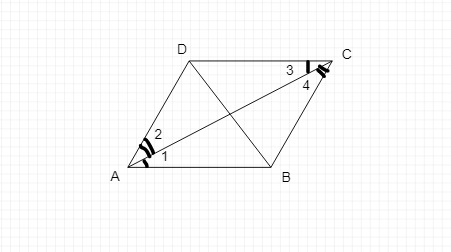
In the parallelogram ABCD, BC || AD and AB || DC. 

AC is the transversal between the parallel lines BC and AD, and it is also a transversal between the parallel lines AB and DC. 

Let the angles formed by these parallel lines and transversal be angles 1, 2, 3 and 4.

Consider ∆ ABC and ∆ CDA, 

∠ 1 = ∠ 3         (since alternate angles are equal)

∠ 2 = ∠ 4         (since alternate angles are equal)

AC = CA          (common side)

So, by the ASA rule ∆ ABC ≅ ∆ CDA. 

Therefore, diagonal AC divides parallelogram ABCD into two congruent triangles ABC and CDA. 

Hence proved.