Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
Construct with ruler and compasses, angles of following measures:
(a) $ 60^{\circ} $
(b) $ 30^{\circ} $
(c) $ 90^{\circ} $
(d) $ 120^{\circ} $
(e) $ 45^{\circ} $
(f) $ 135^{\circ} $
To do:
We have to construct angles of given measures.
Solution:
(a)
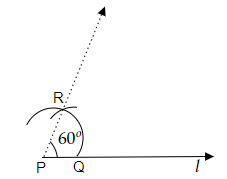
Steps of construction:
(i) Let us draw a line $l$ of any length and mark a point $P$ on the line $l$.
(ii) Now, by taking compasses with any radius let us draw an arc from point $P$ and mark the point of intersection of the arc with the line $l$ as point $R$.
(iii) Now, by taking compasses with the same radius as before let us draw an arc on the previous arc from point $R$ and mark the point of intersection as $S$.
(iv) Now, let us join the points $P$ and $S$.Therefore, the required angle of measure $60^o$ with the line $l$ is formed.
(b)
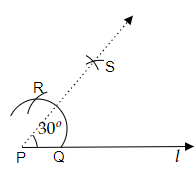
Steps of construction:
(i) Let us draw a line $l$ of any length and mark a point $P$ on the line $l$. Now, by taking compasses with any radius let us draw an arc from point $P$ and mark the point of intersection of the arc with the line $l$ as $Q$.
(ii) Now, by taking compasses with the same radius as before let us draw another arc from point $Q$ and mark the point of intersection of this arc with the previous arc as $R$.
(iii) Now, by taking compasses with the length of radius greater than half of the length from point $Q$ to point $R$ let us draw arcs from point $P$ and $Q$ respectively and let us mark the point of intersection of this arcs to each other as point $S$.
(iv) Now, let us join the point $S$ and point $P$. Therefore, $\overline{PS}$ has the required measure of angle $30^o$ with the line $l$.
(c)
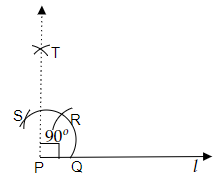
Steps of construction:
(i) Let us draw a line $l$ of any length and mark a point $P$ on the line $l$. Now, by taking compasses with any radius let us draw an arc from point $P$ and mark the point of intersection of this arc with line $l$ as $Q$.
(iii) Now, by taking compasses with the same radius as before let us draw another arc from point $Q$ and mark the point of intersection of this arc with the previous arc as point $R$.
(iv) Now, by taking compasses with the same radius as before let us draw another arc from point $R$ and mark the point of intersection of this arc with the first arc and mark it as point $S$.
(iv) Now, by taking the compasses with the same measure as before let us draw two arcs from point $R$ and point $S$ respectively and mark the point of intersection of these two arcs to each other as point $T$.
(v) Now, let us join point $P$ and point $T$. Therefore, the required angle of measure $90^o$ with the line $l$ is formed.
(d)
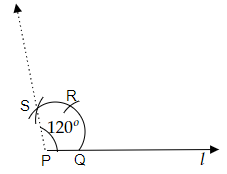
Steps of construction:
(i) Let us draw a line $l$ of any length and mark a point $P$ on the line $l$. Now, by taking compasses with any radius let us draw an arc from point $P$ and mark the point of intersection of the arc with the line $l$ as point $Q$.
(ii) Now, by taking compasses with the same radius as before let us draw another arc from point $Q$ and mark the point of intersection of this arc with the previously drawn arc as point $R$.
(iii) Now, by taking the compasses with the same radius as before let us draw another arc from point $R$ and mark the point of intersection of this arc with the first arc and mark the point of intersection as point $S$.
(iv) Now, join the points $S$ and $P$. Therefore, the required angle of measure $120^o$ with the line $l$ is formed.
(e)
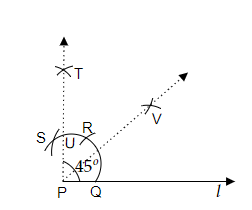
Steps of construction:
(i) Let us draw a line $l$ of any length and mark a point $P$ on the line $l$.
(ii) Now, by taking compasses of any radius let us draw an arc from point $P$ and mark the point of intersection of this arc with line $l$ as point $Q$.
(iii) Now, by taking the compasses with the same radius as before let us draw an arc from point $Q$ and mark the point of intersection of this arc with the previous arc as point $R$.
(iv) Now, by taking compasses with the same radius as before let us draw an arc from point $R$ and mark the point of intersection of this arc with the first arc as point $S$.
(v) Now, by taking compasses with the same radius as before let us draw two arcs from point $R$ and point $S$ respectively and mark the point of intersection of these arcs as point $T$. Let us join point $T$ and point $P$.
(vi) $\overline{PT}$ intersects the first drawn arc at some point let us mark this point as point $U$.
(vii) Now, by taking compasses with the measure of radius greater than half of the length from point $Q$ to $U$ let us draw two arcs from point $Q$ and point $U$ respectively and mark the point of intersection of these arcs to each other as $V$.
(viii) Now, let us join point $P$ and point $V$. Therefore, the required angle of measure $45^o$ with the line $l$ is formed.
(f)
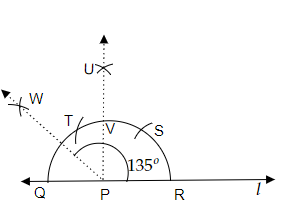
Steps of construction:
(i) Let us draw a line $l$ of any length and mark a point on the line $l$.
(ii) Now, by taking compasses of any radius let us draw a semi-circle from point $P$ and mark the point of intersection of this semi-circle with the line $l$ as point $Q$ and point $R$ respectively.
(iii) Now, by taking compasses with the same radius as before let us draw an arc from point $R$ and mark the point of intersection of this arc with the previous semicircle as point $S$.
(iv) Now, by taking compasses with the radius same as before let us draw an arc from point $S$ and mark the point of intersection of this arc with the semicircle as point $T$.
(v) Now, by taking the same radius as before let us draw two arcs from point $S$ and point $T$ respectively and mark the point of intersection of these arcs to each other as point $U$.
(vi) Now, let us join point $P$ and point $U$ and mark the point of intersection of this $\overline{PU}$ to the semicircle as point $v$.
(vii) Now, by taking compasses with a radius greater than half of the length from point $Q$ to point $V$ let us draw an arc and mark the point of intersection of these arcs to each other as point $W$.
(viii) Now, let us join point $P$ and point $W$. therefore, the required angle of measure $135^o$ with the line $l$ is formed.
34 Views
To Continue Learning Please Login