Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
What is a Metadyne? – Construction, Working, and Applications
Metadyne is a cross-field machine. The cross-field machines are special DC machines having an additional set of brushes on the direct-axis or d-axis. This arrangement of brushes enables the use of armature MMF to provide most of the excitation and achieve high power gains.
Construction and Working of Metadyne
An ordinary DC generator can be converted into a metadyne by providing an additional pair of brushes on the direct-axis or d-axis (see the figure). The brushes lie on the quadrature axis or q-axis are short-circuited and the output of the machine is obtained from the d-axis brushes. The stator consists of a control field winding. A field current $𝐼_{𝑓}$ flows through the control field winding.
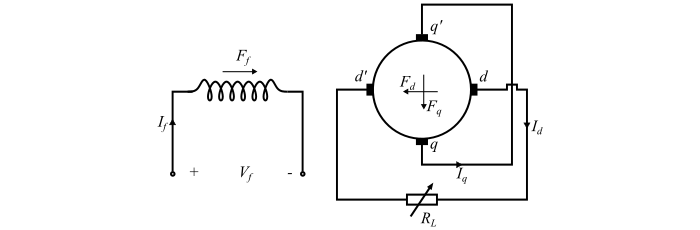
When the rotor of the machine is rotating at a constant speed, the MMF of the control field winding (𝐹𝑓) induces an EMF 𝐸𝑞 between the q-axis brushes qq’. This induced EMF is given by,
$$\mathrm{𝐸_{𝑞} = 𝐾_{𝑞𝑓}𝐼_{𝑓} … (1)}$$
Where, $𝐾_{𝑞𝑓}$ is a constant.
As the brushes qq’ are short circuited, a q-axis armature current (𝐼𝑞) flows and establishes q-axis MMF (𝐹𝑞). Since the impedance of the short-circuited path is very low, therefore, only a small field current (𝐼𝑓) in the control field winding will produce a much larger q-axis armature current. The corresponding flux density wave will be centred on the q-axis. Due to commutator action, this magnetic field is stationary in space. An EMF is induced in the armature by its rotation in the stationary q-axis flux. This generated EMF appears across the daxis brushes dd’ and is given by,
$$\mathrm{𝐸_{𝑑} = 𝐾_{𝑑𝑞}\:𝐼_{𝑞} … (2)}$$
Where, 𝐾𝑑𝑞 is a constant.
Now, if a load of resistance 𝑅𝐿 is connected across the d-axis brushes, the daxis armature current (𝐼𝑑) will flow through the load. This current produces d-axis MMF (𝐹𝑑). According to the Lenz’s law, the d-axis MMF (𝐹𝑑) opposes its cause of production, that is the control field MMF (𝐹𝑓).
Each stage of the generation of voltage produces a current whose magnetic field is 90° ahead of the magnetic flux wave producing the voltage. As there are two stages of the voltage generation, thus the MMF of the d-axis output current is shifted by 90° two times, i.e., 180° and hence opposes the control field MMF (𝐹𝑓). Therefore, the q-axis generated EMF becomes,
$$\mathrm{𝐸_{𝑞} = 𝐾_{𝑞𝑓}\:𝐼_{𝑓} − 𝐾_{𝑞𝑑}𝐼_{𝑑} … (3)}$$
If the magnetic saturation is neglected and the speed of the machine is assumed to be constant, then 𝐾𝑞𝑑 is a constant.
For a given control field MMF (𝐹𝑓) and load resistance, the steady state values of 𝐼𝑑 and 𝐼𝑞 are reached.
From Eqn. (3), it can be seen that any increase in current 𝐼𝑑 decreases the value of EMF 𝐸𝑑. This in turn reduces the current 𝐼𝑑. Therefore, 𝐸𝑑 and 𝐼𝑑 are decreased. Hence for a given value of control field excitation current (𝐼𝑓), the d-axis output current (𝐼𝑑) remains constant over a wide range of load variation. Thus, the above discussion shows that a metadyne behaves as a constant current generator.
Applications of Metadyne
Metadynes were mainly used in the following applications −
To supply DC power to process control motors
To supply the excitation systems of large AC generators
In traction systems and Ward-Leonard speed control systems, etc.
At present, metadynes are not manufactured and are replaced by solid state power amplifiers.
1K+ Views
To Continue Learning Please Login