- Trending Categories
Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
Plot the points $(3, 5)$ and $(-1, 3)$ on a graph paper and verify that the straight line passing through these points also passes through the point $(1, 4)$.
To do:
We have to plot the points $(3, 5)$ and $(-1, 3)$ on a graph paper and verify that the straight line passing through these points also passes through the point $(1, 4)$.
Solution:
$x$ | $3$ | $-1$ |
$y$ | $5$ | $3$ |
Plot the points $A(3, 5)$ and $B(-1, 3)$ on the graph and join them to get the graph.
The above situation can be plotted graphically as below:
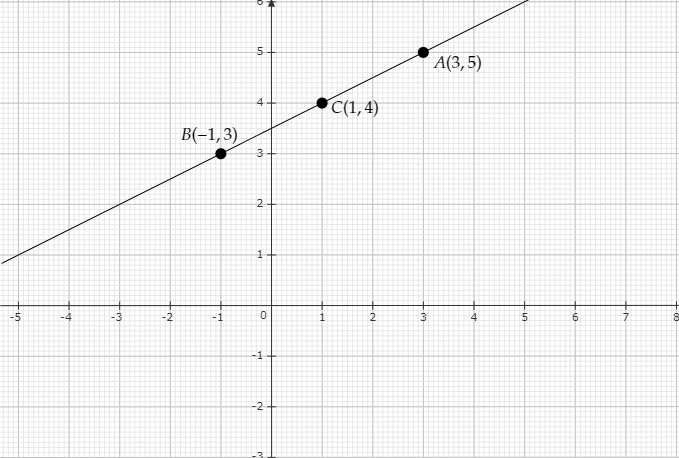
We can observe that $(1, 4)$ lies on the straight line passing through the given points.
- Related Articles
- Plot the following points on the graph paper:$(4, -3)$
- Simplify and verify the result for $p=1$.$4 p^{3} \times 3 p^{4} \times( -p^{5})$"\n
- Plot the following points on the graph paper:$(-3, 2)$
- Write the equal of the line that is parallel to x-axis and passing through the points.$(3, 4)$
- Write the equation of the line that is parallel to y-axis and passing through the points.$(-4, -3)$
- Write the equation of the line that is parallel to y-axis and passing through the points.$(3, 5)$
- The line segment joining the points $(3, -4)$ and $(1, 2)$ is trisected at the points $P$ and $Q$. If the coordinates of $P$ and $Q$ are $(p, -2)$ and $(\frac{5}{3}, q)$ respectively. Find the values of $p$ and $q$.
- Co-ordinates of the point P dividing the line segment joining the points A(1, 3) and B(4, 6), in the ratio 2:1 are:$( A) \ ( 2,4)$ $( B) \ ( 3,\ 5)$ $( C) \ ( 4,\ 2)$ $( D) \ ( 5,\ 3)$
- If the point $A( 0,2)$ is equidistant from the points $B( 3,\ p)$ and $C( p,\ 5)$, find P. Also find the length of AB.
- Find the ratio in which the points $P (\frac{3}{4} , \frac{5}{12})$ divides the line segments joining the points $A (\frac{1}{2}, \frac{3}{2})$ and $B (2, -5)$.
- A point P divides the line segment joining the points$A( 3,\ -5) $ and $B( -4,\ 8)$ such that$\frac{AP}{PB} =\frac{K}{1}$ . if P lies on the line $x+y=0$, then find the value of K.
- A point $P$ divides the line segment joining the points $A (3, -5)$ and $B (-4, 8)$ such that $\frac{AP}{PB} = \frac{k}{1}$. If $P$ lies on the line $x + y = 0$, then find the value of $k$.
- Write the equation of the line that is parallel to x-axis and passing through the points.$(0, 3)$
- The line joining the points $(2, 1)$ and $(5, -8)$ is trisected at the points P and Q. If point P lies on the line $2x – y + k = 0$. Find the value of $k$.
- Simplify and verify for $p=1$ and $q=1$: $( p^{2} \times3p^{3}\times(-8p^{5})$.
Advertisements