Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
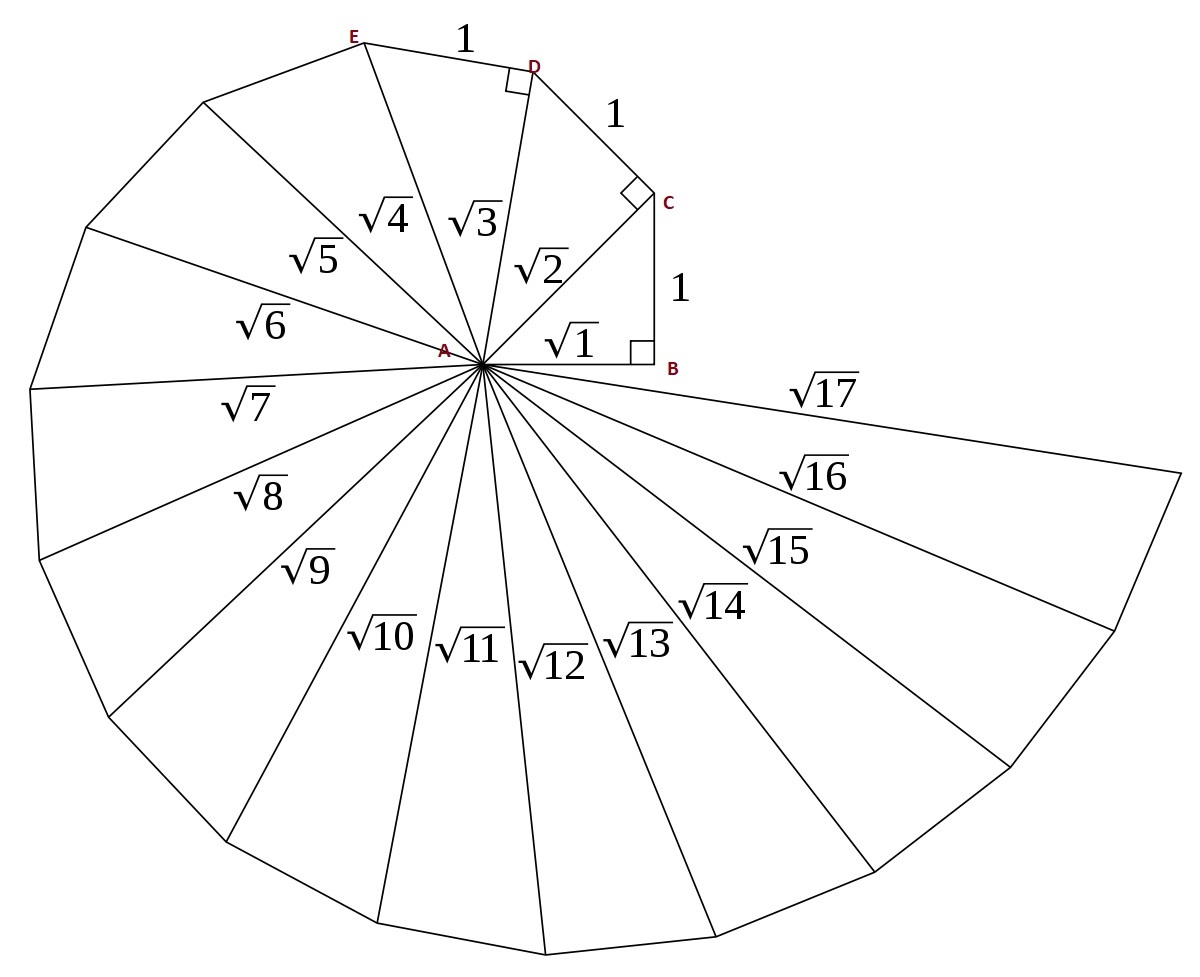
Explain the construction of the square root spiral?
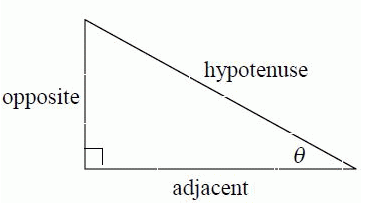
Pythagoras theorem :
Pythagoras theorem is a fundamental relationship among the three sides of a right triangle.
It states that the area of the square whose side is the hypotenuse is equal to the sum of the areas of the squares on the other two sides. $(h^2= a^2+ b^2)$
$h^2= a^2+ b^2$
Where h is the hypotenuse and a, b are the other two sides of the right-angled triangle.
Construction of a Square root spiral:
1. Draw a line segment AB of length 1 unit by taking 1 cm as a unit.
2. Construct a line BX perpendicular to the line segment AB, by using a compass.
3. From B, draw an arc of 1 unit, which cuts BX at C.
4. Join AC.
5. Taking AC as base, draw a perpendicular CY to AC, by using the compass.
6. From C, draw an arc of 1 unit, which cuts CY at D.
7. Join AD.
8. Taking AD as a base, draw a perpendicular DZ to AD, by using the compass.
9. From D, draw an arc of 1 unit, which cuts DZ at E.
10. Join AE.
11. Keep repeating the above process for a sufficient number of times. Then, the figure so obtained is called a square root spiral.
807 Views
To Continue Learning Please Login