A three-wheeler scooter charges Rs. 15 for first kilometer and Rs. 8 each for every subsequent kilometer. For a distance of $x\ km$, an amount of Rs. $y$ is paid. Write the linear equation representing the above information.
Given:
A three-wheeler scooter charges Rs. 15 for first kilometer and Rs. 8 each for every subsequent kilometre. For a distance of $x\ km$, an amount of Rs. $y$ is paid.
To do:
We have to write the linear equation representing the above information.
Solution:
Let the total distance covered be $x\ km$.
Fare for the first kilometer $=1\times 15=Rs.\ 15$
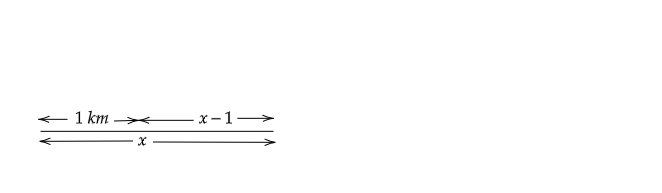
Fare for the subsequent distance$=Rs.\ ( x-1)\times8$
According to the question,
$15+( x-1)8=y$
$\Rightarrow 15+8x-8=y$
$\Rightarrow 8x-y+7=0$
The linear equation representing the given information is $8x-y+7=0$.
Related Articles
- A lending library has a fixed charges for the first three days and an additional charge for each day thereafter. Aarushi paid Rs. 27 for a book kept for seven days. If fixed charges are Rs. x and per day charges are Rs. y. Write the linear equation representing the above information.
- The taxi fare in a city is as follows: For the first kilometre, the fare is Rs 8 and for the subsequent distance it is Rs 5 per \( \mathrm{km} \). Taking the distance covered as \( x \mathrm{~km} \) and total fare as Rs \( y \), write a linear equation for this information, and draw its graph.
- The Taxi Fare In a City is as follows : for the first kilometer the fare Is ₹8 and for the Subsequent distance it is ₹5 Per Kilometer . Taking the distance covered as X Kilometer and total fare as ₹y , write a Linear equation for This Information, And draw it's graph.
- The taxi fare in a city is as follows: For the first kilometre, the fare is \( \mathrm{F} 8 \) and for the subsequent distance it is Rs. 5 per \( \mathrm{km} \). Taking the distance covered as \( x \mathrm{~km} \) and total fare as \( Rs. y \), write a linear equation for this information, and draw its graph.
- The car hire charges in a city comprise of a fixed charges together with the charge for the distance covered. For a journey of 12 km, the charge paid is Rs. 89 and for a journey of 20 km, the charge paid is Rs. 145. What will a person have to pay for travelling a distance of 30 km?
- The taxi fare after each \( \mathrm{km} \), when the fare is Rs 15 for the first \( \mathrm{km} \) and Rs 8 for each additional \( \mathrm{km} \), does not form an AP as the total fare (in Rs) after each \( \mathrm{km} \) is\( 15,8,8,8, \ldots \)Is the statement true? Give reasons.
- In which of the following situations, does the list of numbers involved make an arithmetic progression and why?(i) The taxi fare after each km when the fare is Rs. 15 for the first km and Rs. 8 for each additional km.(ii) The amount of air present in a cylinder when a vacuum pump removes $\frac{1}{4}$ of the air remaining in the cylinder at a time.(iii) The cost of digging a well after every metre of digging, when it costs Rs. 150 for the first metre and rises by Rs. 50 for each subsequent metre.(iv) The amount of money in the account every year, when Rs. 10000 is deposited at compound interest at 8% per annum.
- Taxi service charge Rs. 8 for kilometre and levies a fixed charge of Rs. 50. Write an algebraic expression for the above situation if the taxi is hired from x kilometre.
- A shopkeeper gives books on rent for reading. She takes a fixed charge for the first two days, and an additional charge for each day thereafter. Latika paid Rs. 22 for a book kept for 6 days, while Anand paid Rs. 16 for the book kept for four days. Find the fixed charges and charge for each extra day.
- A lending library has a fixed charge for the first three days and an additional charge for each day thereafter. Saritha paid Rs. 27 for a book kept for seven days, while Susy paid Rs. 21 for the book she kept for five days. Find the fixed charge and the charge for each extra day.
- The salary for a month of an employee is Rs. 4000. The annual salary of the employee is1) RS 480002) RS 240003) RS 120004) RS 8000
- In which of the following situations, does the list of numbers involved make an arithmetic progression and why?The cost of digging a well after every metre of digging, when it costs Rs. 150 for the first metre and rises by Rs. 50 for each subsequent metre.
- A trader recorded below transactions:\na) Started business with cash Rs. 30000\nb) Purchased securities (in cash) in Rs. 9000\nc) Purchased shop for Rs. 30000 (10000 in cash, remaining from loan)\nd) Sold securities for Rs. 1300 (purchased cost = Rs. 900)\ne) Purchased a vehicle (cash) for Rs. 2500\nf) Salary received (in cash) Rs. 5000\ng) Paid Rs.800 for loan and Rs. 400 for interest\nh) Expenses (in cash) paid Rs. 400\ni) Received dividends (in cash) for dividends Rs. 300\nPrepare a table using accounting equation
- The taxi charges in a city consist of a fixed charge together with the charge for the distance covered. For a distance of $10\ km$, the charge paid is $₹\ 105$ and for a journey of $15\ km$, the charge paid is $₹\ 155$. What are the fixed charges and the charge per $km$? How much does a person have to pay for travelling a distance of $25\ km$.
- Tell what is the profit or loss in the following transactions. Also find profit per cent or loss per cent in each case.(a) Gardening shears bought for Rs. 250 and sold for Rs. 325 .(b) A refrigerater bought for \( Rs. 12,000 \) and sold at \( Rs. 13,500 \).(c) A cupboard bought for \( Rs. 2,500 \) and sold at \( Rs. 3,000 \).(d) A skirt bought for \( Rs. 250 \) and sold at \( Rs. 150 \)
Kickstart Your Career
Get certified by completing the course
Get Started
Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies