Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
Induction Motor Speed Control by Pole-Amplitude Modulation
The speed of an induction motor can also be changed with the help of pole-amplitude modulation (PAM) technique. The pole amplitude modulation technique is a flexible method of pole changing which is used in the applications where the speed ratios are other than 2:1. The induction motors designed for the speed control based on pole amplitude modulation technique are called as PAM motors.
To understand the pole amplitude modulation technique, consider the MMF distribution in the air gap of a 3-phase induction motor written as follows
$$\mathrm{𝐹_𝑅 = 𝐹_{𝑚𝑅} \:sin \:𝑝 \theta … (1)}$$
$$\mathrm{𝐹_𝑌 = 𝐹_{𝑚𝑌} \:sin (𝑝 \theta −\frac{2\pi}{3}) … (2)}$$
$$\mathrm{𝐹_𝐵 = 𝐹_{𝑚𝐵} \:sin (𝑝 \theta −\frac{4\pi}{3}) … (3)}$$
Where,
p is the number of pairs of poles,
θ is mechanical angle in radians.
Since the number of turns in each phase winding are equal in a 3-phase induction motor and if the motor is supplied by a balanced 3-phase system, then the maximum values of the MMFs in all the three phases is the same.
Now, if three modulating MMF waves of amplitude F which are displaced from each other by (2π/3) radians are used to modulate the MMF waves of eqns. (1), (2) & (3), then the FmR, FmY and FmB can be written as,
$$\mathrm{𝐹_{𝑚𝑅} = 𝐹 sin 𝑘 \theta … (4)}$$
$$\mathrm{𝐹_{𝑚𝑌} = 𝐹 sin(𝑘 \theta − \propto) … (5)}$$
$$\mathrm{𝐹_{𝑚𝐵} = 𝐹 sin(𝑘 \theta − 2\propto) … (6)}$$
Where,
k is the number of modulating cycles in one complete perimeter of the motor,
∝ = (± 2π/3) radian.
Substituting the eqns. (4), (5) & (6) in the eqns. (1), (2) & (3) gives,
$$\mathrm{𝐹_𝑅 = 𝐹 sin 𝑘 \theta . sin 𝑝 \theta … (7)}$$
$$\mathrm{𝐹_𝑌 = 𝐹 sin(𝑘 \theta − 𝛼) . sin (𝑝 \theta −\frac{2\pi}{3}) … (8)}$$
$$\mathrm{𝐹𝐵 = 𝐹 sin(𝑘 \theta − 2\propto) . sin (𝑝 \theta −\frac{4\pi}{3}) … (9)}$$
Using trigonometric identities, eqns. (7), (8) & (9) can also be written as,
$$\mathrm{𝐹_𝑅 =\frac{𝐹}{2}[cos(𝑝 − 𝑘) \theta − cos(𝑝 + 𝑘) \theta] … (10)}$$
$$\mathrm{𝐹_𝑌 ={\frac{𝐹}{2}{cos [(𝑝 − 𝑘) \theta −\frac{2\pi}{3}+ 𝛼] − cos [(𝑝 + 𝑘) \theta −\frac{2\pi}{3}− \propto]}} … (11)}$$
$$\mathrm{𝐹_𝐵 ={\frac{𝐹}{2}{cos [(𝑝 − 𝑘) \theta −\frac{4\pi}{3}+ 2\propto] − cos [(𝑝 + 𝑘) \theta −\frac{4\pi}{3}− 2\propto]}} … (12)}$$
Hence, from eqns. (10), (11) & (12), it is clear that by modulating the amplitudes of the MMFs in the 3-phase induction motor having p-pairs of poles, the two sets of 3-phase MMFs with (p-k) and (p+k) poles can be produced. These two sets of poles will produce torques in opposite directions.
In order to obtain unidirectional steady torque, one of these pole pairs must be supressed and the other pole pair should be retained. For the pole amplitude modulation, a rectangular space MMF wave of unit amplitude and of period equal to the length of the stator periphery of the motor is used.
To obtain the desired modulation, two methods of connections are used, which are given as follows −
- Coil inversion
- Coil inversion and omission
In both the methods, the windings of each phase are divided in two parts.
Coil Inversion Method
In the method of coil inversion, the current through the latter half of winding in each phase is reversed. The figure shows the principle of pole amplitude modulation by the coil inversion method.
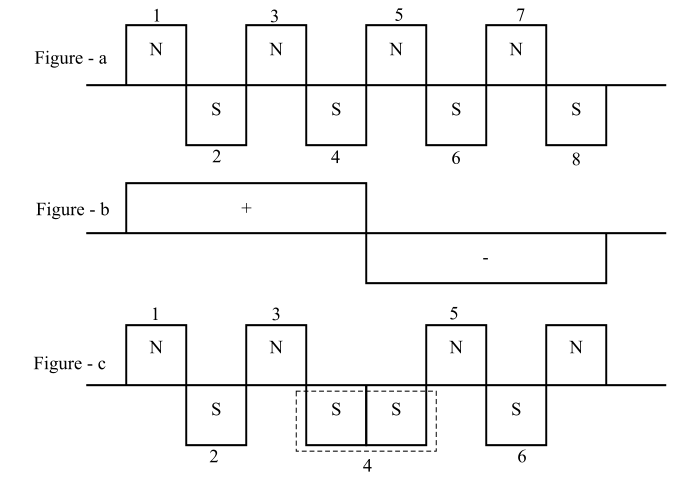
Here, Figure-(a) shows the MMF wave of a stator wound for 8-poles. Figure-(b) shows a 2-pole modulating wave. The negative half cycle of the modulating wave reverses the polarities of the stator poles 5, 6, 7 and 8. Figure-(c) shows the resultant modulated wave which has 6 poles.
Coil Inversion and Omission Method
In the method of coil inversion and omission, a part of the winding is omitted from each half of the winding and the remaining portion of the winding is then reversed. The figure below shows the principle of pole amplitude modulation by coil inversion and omission
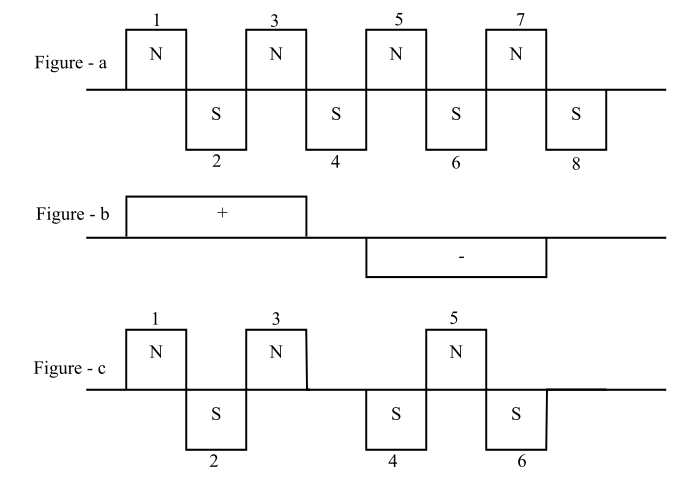
Here, Figure-(a) shows the MMF wave of a stator wound for 8-poles. Figure-(b) shows a 2-pole modulating wave. The fourth and eighth coils are omitted and the fifth, sixth and seventh coils are reversed with respect to the first three coils. Thus, Figure-(c) shows the resultant modulated wave having 6-poles.
In a 3-phase induction motor, by the proper choice of series or parallel connection between the coil groups of each phase and star or delta connection between the phases, the speed control can be obtained with constant-torque operation, constant-power operation or variable-torque operation.
The pole amplitude modulation (PAM) technique for speed control is mainly used in pump, blower and fan drives.
890 Views
