Force on a Current Carrying Conductor in a Magnetic Field

When a current carrying conductor is placed at right angles to a magnetic field, it is found that a force acts on the conductor in a direction perpendicular to the direction of both the magnetic field and the current.

Explanation

Consider a straight conductor carrying a current of I amperes. If the magnetic flux density is B, the effective length of the conductor is l and θ is the angle which the conductor makes with the direction of the magnetic field.

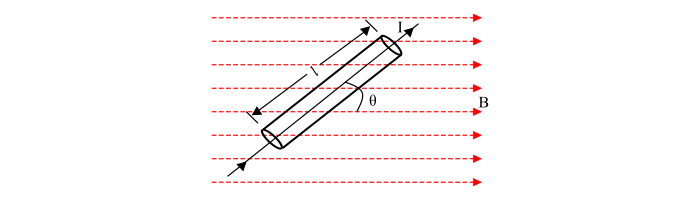
It has been found by experiments that the magnitude of the force (F) acting on the conductor is directly proportional to −

• Magnetic flux density (B),

• Current through the conductor (I), and

• Sine of the angle θ i.e. sinθ.

Therefore,

$$\mathrm{\mathit{F\propto BIl\:sinθ}}$$

$$\mathrm{\Longrightarrow \mathit{F= K BIl\:sinθ}}$$

Where, k is constant of proportionality and its value is unity in SI units. Thus,

$$\mathrm{\mathit{F = BIl\:sinθ}\:\:\:\:\:\:...(1)}$$

• Case 1 − When θ = 0° or 180° then sin θ = 0, hence,

  $$\mathrm{\mathit{F }= 0\:\:\:\:\:\:...(2)}$$

• Case 2 − When θ = 90°, then sin θ = 1, hence,

  $$\mathrm{\mathit{F=BIl}\:\:\:\:\:\:...(3)(𝑖i.e F is maximum)}$$

Numerical Example

A straight wire 0.5 m long carries a current of 150 A and lies at an angle of 60° to a uniform magnetic field of 2.5 Wb/m².Find the mechanical force on the conductor when (a) it lies in the given position, (b) it lies in a position such that it is at right angles to the magnetic field.

Solution

• When conductor lies at 60° to the magnetic field

    $$\mathrm{\mathit{F=BIl\:sinθ}=2.5\times150\times0.5\times sin60=162.38N}$$

• When conductor lies at right angles to the magnetic field

  $$\mathrm{\mathit{F=BIl}=2.5\times150\times0.5=187.5N}$$