Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
Area of the largest triangle that can be inscribed within a rectangle?
A rectangle is a quadrilateral that has opposite side equal and parallel. The adjacent side are at 90o. And a triangle is a closed figure with three sides.
The largest triangle inscribed within a rectangle. Has its base equal to the length of the rectangle and height of the triangle is equal to the breadth of the rectangle.
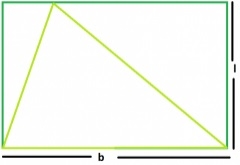
Area = (½)*l*b
Area of largest triangle inscribed in a rectangle = (½)*l*b
Program to calculate the area of the largest triangle inscribed in a rectangle −
Example Code
#include <stdio.h>
int main(void) {
int l = 10, b = 9;
float area ;
area = (float)((l*b)/2);
printf("Area of largest triangle inscribed in a rectangle of
length %d and breadth %d is %f",l,b,area);
return 0;
}Output
Area of largest triangle inscribed in a rectangle of length 10 and breadth 9 is 45.000000
Updated on: 30-Jul-2019
154 Views
Advertisements