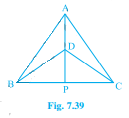
$ \triangle \mathrm{ABC} $ and $ \triangle \mathrm{DBC} $ are two isosceles triangles on the same base $ B C $ and vertices $ A $ and $ D $ are on the same side of $ \mathrm{BC} $ (see Fig. 7.39). If $ \mathrm{AD} $ is extended to intersect $ \mathrm{BC} $ at $ \mathrm{P} $, show that
(i) $ \triangle \mathrm{ABD} \equiv \triangle \mathrm{ACD} $
(ii) $ \triangle \mathrm{ABP} \cong \triangle \mathrm{ACP} $
(iii) $ \mathrm{AP} $ bisects $ \angle \mathrm{A} $ as well as $ \angle \mathrm{D} $.
(iv) AP is the perpendicular bisector of $ \mathrm{BC} $.
"

Given:

$\triangle ABC$ and $\triangle DBC$ are two isosceles triangles on the same base $BC$ and vertices $A$ and $D$ are on the same side of $BC$. If $AD$ is extended to intersect $BC$ at $P$.

To do:

We have to show:

(i) $\triangle ABD \cong \triangle ACD$

(ii) $\triangle ABP \cong \triangle ACP$
(iii) $AP$ bisects $\angle A$ as well as $\angle D$.
(iv) $AP$ is the perpendicular bisector of $BC$.

Solution:

(i) We know that,

The side-Side-Side congruence rule states that if three sides of one triangle are equal to three corresponding sides of another triangle, then the triangles are congruent.

Let us consider $\triangle ABD$ and $\triangle ACD$

Given,

$\triangle ABC$ and $\triangle DBC$ are isosceles triangles,

This implies,

$AB=AC$ and $BD=CD$

Since $AD$ is the common side 

$AD=AD$

Therefore,

$\triangle ABD \cong \triangle ACD$

(ii) Let us consider $\triangle ABP$ and $\triangle ACP$

Given,

$\triangle ABC$ is isosceles,

This implies,

$AB=AC$

Since $AP$ is the common side

We get,

$AP=AP$

We also know 

From corresponding parts of congruent triangles: If two triangles are congruent, all of their corresponding angles and sides must be equal.

Therefore,

$\angle PAB=\angle PAC$.

Therefore, 

According to the Rule of Side-Angle-Side Congruence:

Triangles are said to be congruent if any pair of corresponding sides and their included angles are equal in both triangles.

 Hence, $\triangle ABP \cong \triangle ACP$.

(iii) We know that,

From corresponding parts of congruent triangles: If two triangles are congruent, all of their corresponding angles and sides must be equal.

Therefore,

$\angle PAB=\angle PAC$   (since $\triangle ABD \cong \triangle ACD$)

Given,

$AP$ bisects $\angle A$...(i)

Let us consider $\triangle BPD$ and $\triangle CPD$

We also know that,

The side-Side-Side congruence rule states that if three sides of one triangle are equal to three corresponding sides of another triangle, then the triangles are congruent.

Since $PD$ is the common side.

We get, $PD=PD$

As $\triangle DBC$ is isosceles We get,

$BD=CD$

and by CPCT we know that as $\triangle ABP \cong \triangle ACP$

Therefore we get,

$\triangle BPD \cong \triangle CPD$

Hence, $\angle BDP=\angle CDP$ by CPCT....(ii)

Now, by comparing (i) and (ii) We can say that $AP$ bisects $\angle A$ as well as $\angle D$.

(iv) Let us consider $\triangle BPD$ and $\triangle CPD$ 

We know that,

From corresponding parts of congruent triangles: If two triangles are congruent, all of their corresponding angles and sides must be equal.

Therefore,

$\angle BPD=\angle CPD$

and $BP=CP$ ....(i)

We also know that,

The sum of the angles of a straight line is $180^o$

$\angle BPD+\angle CPD=180^o$

Since $\angle BPD=\angle CPD$

We get,

$2\angle BPD=180^o$

$\angle BPD=\frac{180^o}{2}$

$\angle BPD=90^o$.....(ii)

From (i) and (ii) we can say that,

$AP$ is the perpendicular bisector of $BC$.