The values of current (I) flowing through a given resistor of resistance (R), for the corresponding values of potential difference (V) across the resistor are as given below :

V (Volts)	0.5	1.0	1.5	2.0	2.5	3.0	4.0	5.0
I (Amperes)	0.1	0.2	0.3	0.4	0.5	0.6	0.8	1.0

Plot a graph between current (I) and potential difference (V) and determine the resistance (R) of the resistor.

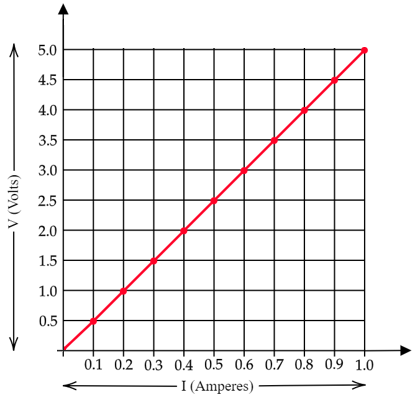
Resistance of the resistor (R) = Slope of the above graph

Choose any two points P and Q on the graph,

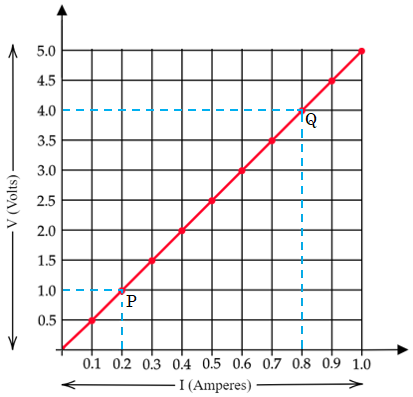
Resistnce of the resistor (R) = Slope of line $PQ=\frac{4-1}{0.8-02}=\frac{3}{0.6}=\frac{30}{6}=5\Omega $

Alternate Solution

We know that-

$R=\frac{\Delta V}{\Delta I}$

Where, 

$R$ = Resistance

$V$ = Potential difference or Voltage

$I$ = Current

$\Delta $ = shows change

Putting the given values we get-

$R=\frac{3-2}{0.6-0.4}$

$R=\frac{1}{0.2}$

$R=\frac{10}{2}$

$R=5\Omega $

NOTE: For every point on the graph, the resistance will remain the same.

Tutorialspoint

Simply Easy Learning

Updated on: 10-Oct-2022

162 Views

Kickstart Your Career

Get certified by completing the course

Get Started