The annual profits earned by 30 shops of a shopping complex in a locality give rise to the following distribution:

Profit (in lakhs in Rs)	Number of shops (frequency)
More than or equal to 5	30
More than or equal to 10	28
More than or equal to 15	16
More than or equal to 20	14
More than or equal to 25	10
More than or equal to 30	7
More than or equal to 35	3

Draw both ogives for the above data and hence obtain the median.

Given:

We have to draw both ogives for the above data and hence obtain the median.

Solution:

We first prepare the cumulative  frequency distribution table by more than method as given below:

Represent profits(in lakhs) along X-axis and cumulative frequency along Y-axis.

Plot the points (5, 30), (10, 28), (15, 16), (20, 14), (25, 10), (30, 7) and (35, 3) on the graph and join them in free hand to get a more than ogive.

We first prepare the cumulative  frequency distribution table by less than method as given below:

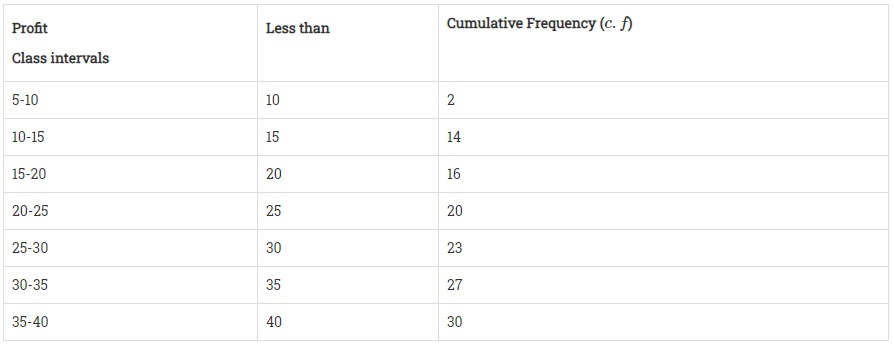
Represent profits(in lakhs) along X-axis and cumulative frequency along Y-axis.

Plot the points (10, 3), (15, 7), (20, 10), (25, 14), (30, 16), (35, 28) and (40, 30) on the graph and join them in a free hand to get a less than ogive.

Total number of days $N = 30$

This implies,

$\frac{N}{2} = \frac{30}{2}=15$

Draw a line parallel to X-axis at the point of intersection of both ogives, which further intersect at (0, 12) on Y-axis.

Draw a line perpendicular to X-axis at point of intersection of both ogives, which further intersect at (17.50, 0) on X-axis.

Therefore, 17.50 is the required median using ogives.

Hence, the median is 17.50 lakhs.

Tutorialspoint

Simply Easy Learning

Updated on: 10-Oct-2022

52 Views

Kickstart Your Career

Get certified by completing the course

Get Started