$S$ and $T$ are points on sides $PR$ and $QR$ of $∆PQR$ such that $\angle P = \angle RTS$. Show that $∆RPQ \sim ∆RTS$.
Given:
$S$ and $T$ are points on sides $PR$ and $QR$ of $∆PQR$ such that $\angle P = \angle RTS$.
To do:
We have to show that $∆RPQ \sim ∆RTS$.
Solution:
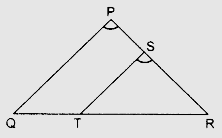
In $\triangle RPQ$ and $\triangle RTS$,
$\angle P=\angle RTS$
$\angle P=\angle R$
Therefore, by AA criterion,
$\Delta RPQ \sim \Delta RTS$
Hence proved.
Related Articles
- In $∆ABC$, $\angle A=30^{\circ},\ \angle B=40^{\circ}$ and $\angle C=110^{\circ}$In $∆PQR$, $\angle P=30^{\circ},\ \angle Q=40^{\circ}$ and $\angle R=110^{\circ}$. A student says that $∆ABC ≅ ∆PQR$ by $AAA$ congruence criterion. Is he justified? Why or why not?
- CD and GH are respectively the bisectors of $\angle ACB$ and $\angle EGF$ such that D and H lie on sides AB and FE of $∆ABC$ and $∆EFG$ respectively. If $∆ABC \sim ∆FEG$, show that (i) \( \frac{\mathrm{CD}}{\mathrm{GH}}=\frac{\mathrm{AC}}{\mathrm{FG}} \)>(ii) \( \triangle \mathrm{DCB} \sim \triangle \mathrm{HGE} \)>(iii) \( \triangle \mathrm{DCA} \sim \triangle \mathrm{HGF} \)
- Construct the right angled $∆PQR$, where $m\angle Q = 90^{\circ},\ QR = 8cm$ and $PR = 10\ cm$.
- If $∆DEF ≅ ∆BCA$, write the part(s) of $∆BCA$ that correspond to$(i).\ \angle E$$(ii).\ \overline{EF}$$(iii).\ \angle F$$(iv).\ \overline{DF}$
- ∆ABC ~ ∆LMN. In ∆ABC, AB = 5.5 cm, BC = 6 cm, CA = 4.5 cm. Construct ∆ABC and ∆LMN such that $\frac{BC}{MN} \ =\ \frac{5}{4}$.
- In the given figure, $\frac{QR}{QS}=\frac{QT}{PR}$ and $\angle 1 = \angle 2$. show that $∆PQR \sim ∆TQR$."
- $E$ is a point on the side $AD$ produced of a parallelogram $ABCD$ and $BE$ intersects $CD$ at $F$. Show that $∆ABE \sim ∆CFB$.
- Construct $∆DEF$ such that $DE = 5\ cm$, $DF = 3\ cm$ and $\angle EDF = 90^{\circ}$.
- D, E and F are respectively the mid-points of sides AB, BC and CA of $∆ABC$. Find the ratio of the areas of $∆DEF$ and $∆ABC$.
- Construct $∆ABC$ such that $AB=2.5\ cm$, $BC=6\ cm$ and $AC=6.5\ cm$. Measure $\angle B$.
- If \( \angle A \) and \( \angle P \) are acute angles such that \( \tan A=\tan P \), then show that \( \angle A=\angle P \).
- Draw $∆PQR$ with $PQ=4\ cm$, $QR=3.5\ cm$ and $PR=4\ cm$. What type of triangle is this?
- Construct $∆PQR$ if $PQ = 5\ cm$, $m\angle\ PQR = 105^{\circ}$ and $m\angle QRP = 40^{\circ}$. [Hint: Recall angle-sum property of a triangle.]
Kickstart Your Career
Get certified by completing the course
Get Started
Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies