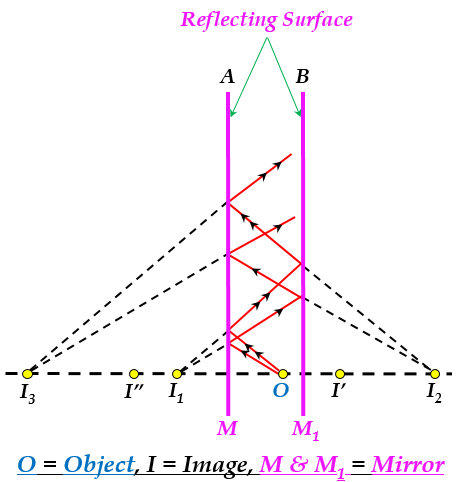
Two plane mirrors are arranged such that their reflecting surface are parallel and face each other. When an object is placed between them the number of images formed are ____________.
(1) 2 (2) infinite (3) 3 (4) 4
37811"
The correct answer is option (2) infinite.
When two plane mirrors facing each other parallelly (i.e., aligned at 0° angle), forms an infinite number of images of an object kept in between them.
Related Articles
- How many images are formed, when two plane mirrors are placed parallel and facing each other?
- Show that the quadrilateral whose vertices are $(2, -1), (3, 4), (-2, 3)$ and $(-3, -2)$ is a rhombus.
- Prove that the points $(-4, -1), (-2, -4), (4, 0)$ and $(2, 3)$ are the vertices of a rectangle.
- Show that the points $(-4, -1), (-2, -4), (4, 0)$ and $(2, 3)$ are the vertices points of a rectangle.
- Show that the points $A( 2,\ -1),\ B( 3,\ 4),\ C( -2,\ 3)$ and $D( -3,\ -2)$ are the vertices of a rhombus.
- Without using formula, show that points $( -2,\ -1),\ ( 4,\ 0),\ ( 3,\ 3)$ and $( -3,\ 2)$ are the vertices of a parallelogram.
- Two particles are placed at some distance from each other. If, keeping the distance between them unchanged, the mass of each of the two particles is doubled, the value of gravitational force between them will become:(a) 1/4 times(b) 1/2 times(c) 4 times(d) 2 times
- Find the area of quadrilateral ABCD, whose vertices are: $A( -3,\ -1) ,\ B( -2,\ -4) ,\ C( 4,\ -1)$ and$\ D( 3,\ 4) .$
- Show that $A (-3, 2), B (-5, -5), C (2, -3)$ and $D (4, 4)$ are the vertices of a rhombus.
- How many images of a candle will be formed if it is placed between two parallel plane mirrors separated by 40 cm?
- Sum of the series 1 + (1+2) + (1+2+3) + (1+2+3+4) + ... + (1+2+3+4+...+n) in C++
- The number of pairs of two digit square numbers, the sum or difference of which are also square numbers is(1) 0(2) 1(3) 2(4) 3
- Observe the following pattern$1=\frac{1}{2}\{1 \times(1+1)\}$$1+2=\frac{1}{2}\{2 \times(2+1)\}$$1+2+3=\frac{1}{2}\{3 \times(3+1)\}$$1+2+3+4=\frac{1}{2}\{4 \times(4+1)\}$and find the values of each of the following:(i) $1 + 2 + 3 + 4 + 5 +….. + 50$(ii)$31 + 32 +… + 50$
- Count number of trailing zeros in (1^1)*(2^2)*(3^3)*(4^4)*.. in C++
- Following data gives the number of children in 41 families:$1, 2, 6, 5, 1, 5, 1, 3, 2, 6, 2, 3, 4, 2, 0, 0, 4, 4, 3, 2, 2, 0, 0, 1, 2, 2, 4, 3, 2, 1, 0, 5, 1, 2, 4, 3, 4, 1, 6, 2, 2.$Represent it in the form of a frequency distribution.
Kickstart Your Career
Get certified by completing the course
Get Started
Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies