The table given below shows the population (in thousands) of men and women in a village in different years.

Year	2013	2014	2015	2016	2017
Number of men	11.5	12.4	13	13.8	14.2
Number of women	10.5	11.7	13	14.2	15.0

Draw two line graphs for the above data

Let us take the years on x-axis and population on the y-axis.

Taking the scale 1 unit $=$ population of 500 on the y-axis and 1 unit $=$ 2 years on the x-axis.
 

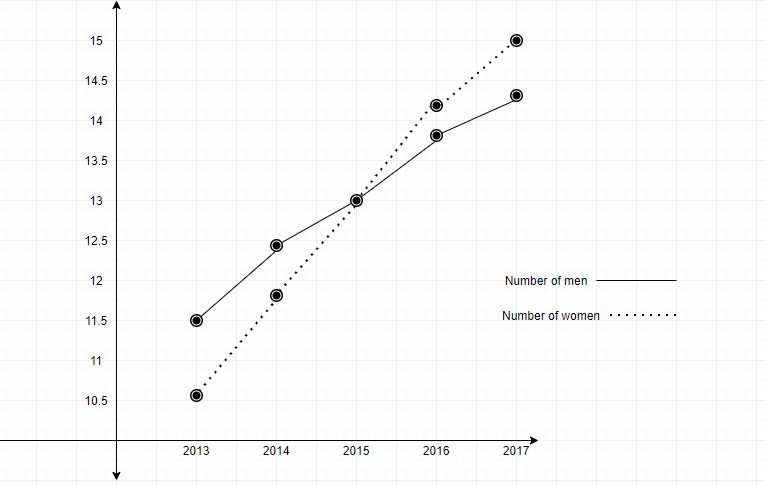
(i) The ratio of the male population and female population in the year $2013=\frac{11.5\times1000}{10.5\times1000}=\frac{115}{105}=\frac{23}{21}$.

(ii) The ratio of the total population of the village in 2015 to that in 2017 $=\frac{(13+13)\times1000}{(14.2+15)\times1000}=\frac{26}{29.2}=\frac{130}{146}=\frac{65}{73}$

(iii)  The percentage increase in the population of women in 2017 as compared to that in 2014$=\frac{(15-11.7)\times1000}{11.7\times1000}\times100 \%=\frac{33}{117}\times100 \%=\frac{11}{39}\times100 \%=28.21 \%$

(iv) Percentage increase in the men from 2013 to 2014$=\frac{(12.4-11.5)\times1000}{11.5\times1000}\times100 \%=\frac{9}{115}\times100 \%=\frac{900}{115} \%=7.82 \%$

Percentage increase in the men from 2014 to 2015$=\frac{(13-12.4)\times1000}{12.4\times1000}\times100 \%=\frac{6}{124}\times100 \%=\frac{600}{124} \%=4.84 \%$

Percentage increase in the men from 2015 to 2016$=\frac{(13.8-13)\times1000}{13\times1000}\times100 \%=\frac{8}{130}\times100 \%=\frac{80}{13} \%=6.15 \%$

Percentage increase in the men from 2016 to 2017$=\frac{(14.2-13.8)\times1000}{13.8\times1000}\times100 \%=\frac{4}{138}\times100 \%=\frac{400}{138} \%=2.90 \%$

Between 2013 and 2014 the percentage increase in the number of men is maximum.