The horizontal distance between two trees of different heights is $ 60 \mathrm{~m} $. The angle of depression of the top of the first tree when seen from the top of the second tree is $ 45^{\circ} $. If the height of the second tree is $ 80 \mathrm{~m} $, find the height of the first tree.

Given:

The horizontal distance between two trees of different heights is \( 60 \mathrm{~m} \).

The angle of depression of the top of the first tree when seen from the top of the second tree is \( 45^{\circ} \).

The height of the second tree is \( 80 \mathrm{~m} \)

To do:

We have to find the height of the first tree.

Solution:

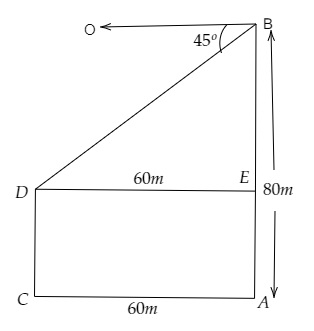
Let $AB$ and $CD$ be the two trees, the height of the second tree $AB=80\ m$.

Let the height of first pole $CD=h\ m$

Distance between the two poles, $AC = 60\ m$

From the figure,

$AC=DE= 60\ m$ and $AE=CD=h$ and $BE = 80-h\ m$

In $\vartriangle DBE$,

$tan45^{o}=\frac{BE}{DE}$

$\Rightarrow 1 =\frac{80-h}{60}$

$\Rightarrow 60=80-h$

$\Rightarrow h=80-60$

$\Rightarrow h=20\ m$

Therefore, the height of the first tree is $20\ m$.  

Tutorialspoint

Simply Easy Learning

Updated on: 10-Oct-2022

60 Views

Kickstart Your Career

Get certified by completing the course

Get Started