Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
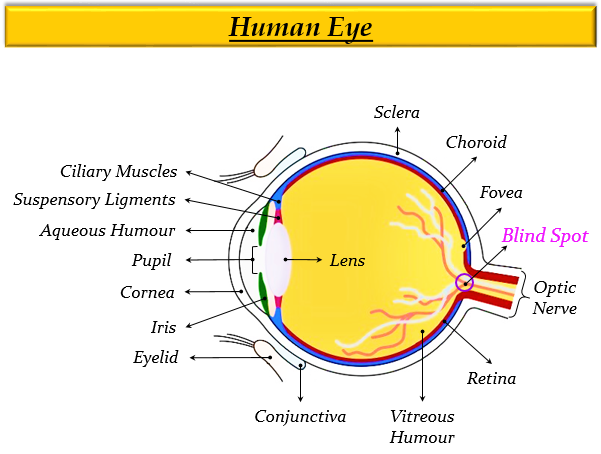
Name the part of our eyes that helps us to focus near and distant objects in quick succession.
Ciliary muscles are the part of the eyes that helps us to focus near and distant objects in quick succession.
Explanation
The ciliary muscle is a ring of smooth muscle in the eye's middle layer that controls accommodation for viewing objects at varying distances which means, it changes the shape of the lens to focus on near or distant objects.
Therefore, when looking at the distant object, the ciliary muscles become fully relaxed which result in a decrease in the thickness of the lens, and the eye lens very thin. In this position, its focal length is maximum, and converging is minimum to focus the parallel rays on the retina.
While, when looking at the nearby object, the ciliary muscles get stretched which result in an increase in the thickness of the lens, and the eye lens thick. In this position, its focal length is minimum and converging is maximum to focus
the diverging rays on the retina.
274 Views
