"
">

In the figure two straight lines $ \mathrm{AB} \& \mathrm{CD} $ intersect at $ \mathrm{O} $. If $ \angle \mathrm{COT}=60^{\circ} $, find $ \mathrm{a}, \mathrm{b}, \mathrm{c} $."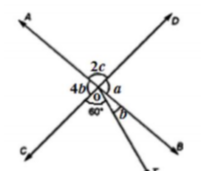

Academic Mathematics NCERT Class 9

Given:

Two straight lines \( \mathrm{AB} \& \mathrm{CD} \) intersect at \( \mathrm{O} \).

\( \angle \mathrm{COT}=60^{\circ} \),
To do:

We have to find \( \mathrm{a}, \mathrm{b}, \mathrm{c} \).

Solution:

We know that,

Vertically opposite angles are equal.

Sum of the angles on a straight line is $180^o$.

Sum of the angles about a point is $360^o$.
Therefore,

$a=4b$....(i)

$4b+60^o+b=180^o$

$\Rightarrow 5b=180^o-60^o$

$\Rightarrow 5b=120^o$

$\Rightarrow b=\frac{120^o}{5}$

$\Rightarrow b=24^o$

$a=4(24^o)$

$=96^o$

$4b+2c=180^o$

$4(24^o)+2c=180^o$

$96^o+2c=180^o$

$2c=180^o-96^o$

$2c=84^o$

$c=\frac{84^o}{2}$

$c=42^o$

Therefore, $\mathrm{a}=96^o, \mathrm{b}=24^o, \mathrm{c}=42^o$.

Simply Easy Learning

Updated on: 10-Oct-2022

49 Views

Kickstart Your Career

Get certified by completing the course

Advertisements