In the figure, $ P A $ and $ P B $ are tangents to the circle from an external point $ P $. $ C D $ is another tangent touching the circle at $ Q $. If $ PA=12\ cm, QC=QD=3\ cm, $ then find $ P C+P D $."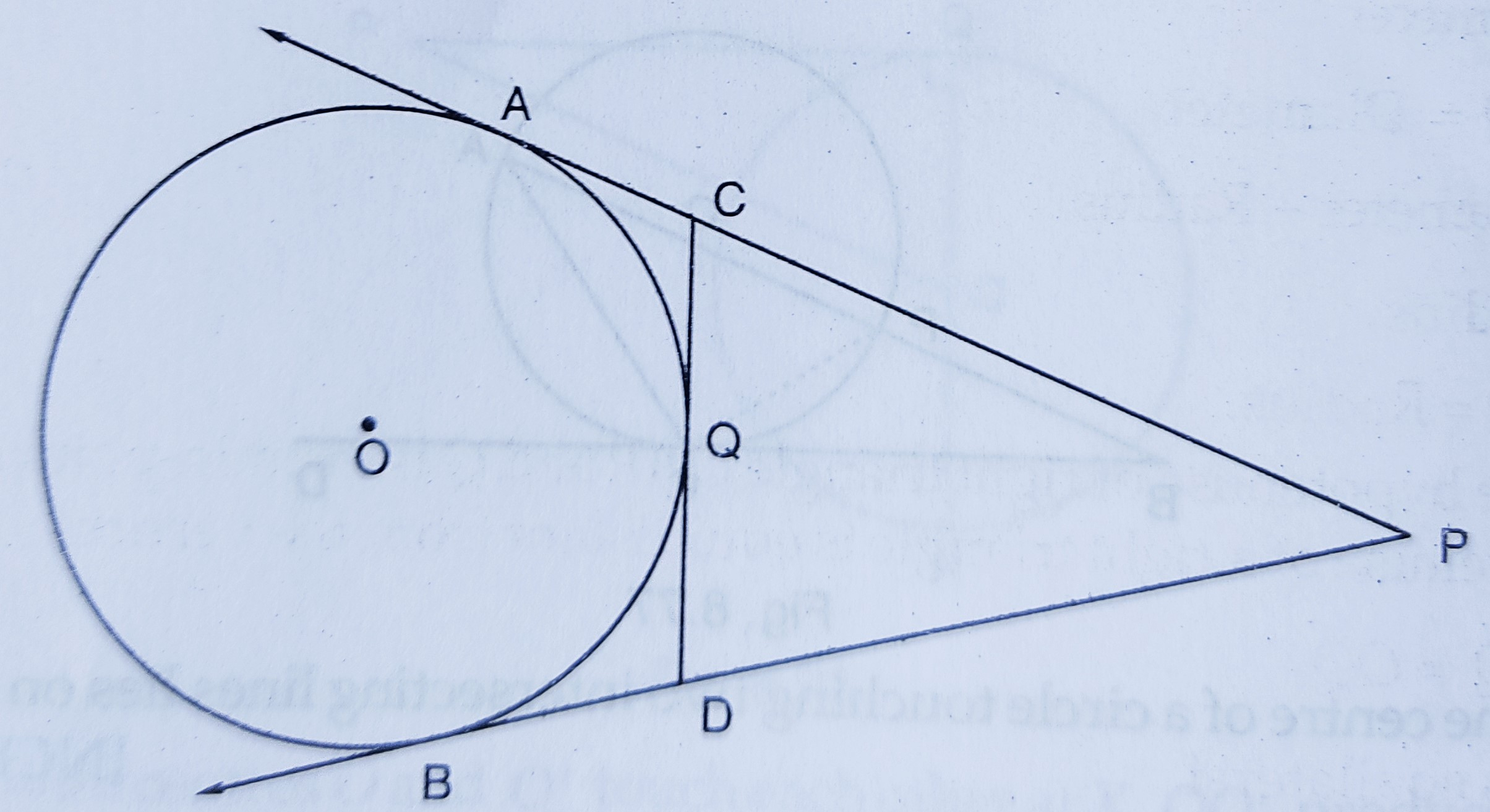

Given:

In the figure, \( P A \) and \( P B \) are tangents to the circle from an external point \( P \). \( C D \) is another tangent touching the circle at \( Q \).

\( PA=12\ cm, QC=QD=3\ cm. \)

To do:
We have to find \( P C+P D \).

 Solution:

We know that,

Tangents drawn from an external point are equal.

This implies,

$PA = PB = 12\ cm$.....…(i)

$QC = AC = 3\ cm$......(ii)

$QD = BD = 3\ cm$.......(iii)

Therefore,

$PC + PD = (PA - AC) + (PB - BD)$

$= (12 - 3) + (12 - 3)$    [From (i), (ii) and (iii)]

$= 9 + 9$

$= 18\ cm$

Therefore, \( P C+P D = 18\ cm \).