In the figure, $ B D C $ is a tangent to the given circle at point $ D $ such that $ B D=30 \mathrm{~cm} $ and $ C D=7 \mathrm{~cm} $. The other tangents $ B E $ and $ C F $ are drawn respectively from $ B $ and $ C $ to the circle and meet when produced at $ A $ making $ B A C $ a right angle triangle. Calculate $ A F $."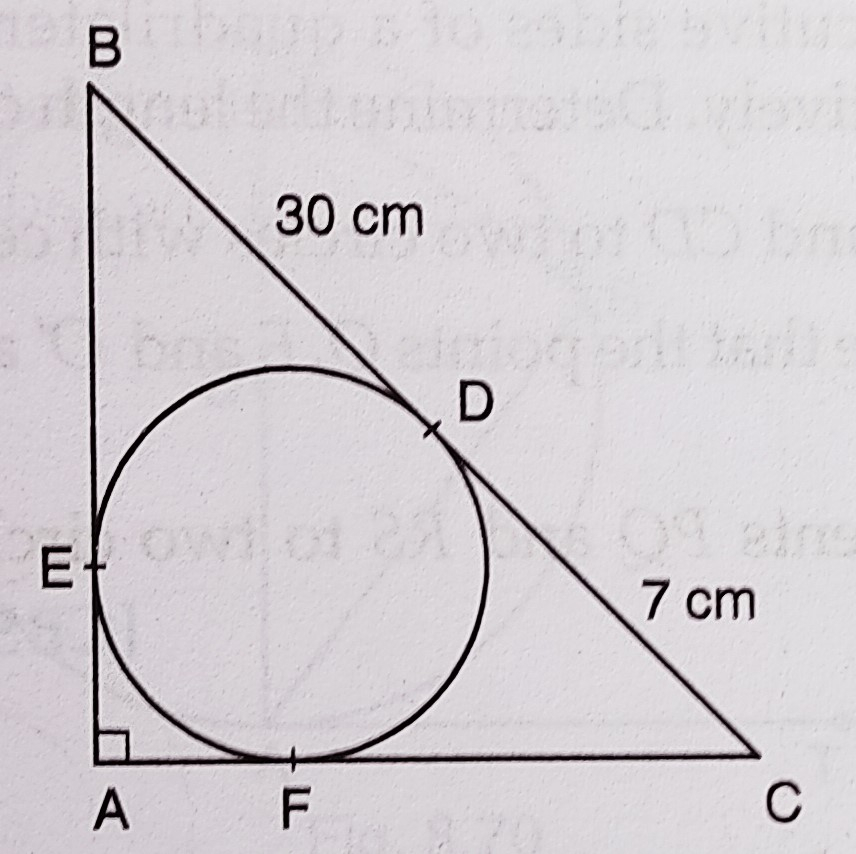

Given:

In the figure, \( B D C \) is a tangent to the given circle at point \( D \) such that \( B D=30 \mathrm{~cm} \) and \( C D=7 \mathrm{~cm} \).

The other tangents \( B E \) and \( C F \) are drawn respectively from \( B \) and \( C \) to the circle and meet when produced at \( A \) making \( B A C \) a right angle triangle.

To do:

We have to calculate \( A F \).

Solution:

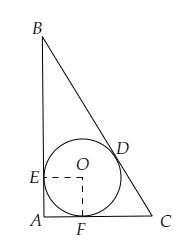
$AB, BC$ and $AC$ are tangents to the circle at $E, D$ and $F$.

$BD = 30\ cm$ and $DC = 7\ cm$ and $\angle BAC = 90^o$  Tangents drawn from an exterior point to a circle are equal in length. 

This implies,

$BE = BD = 30\ cm $

$FC = DC = 7\ cm$

Let $AE = AF = x$

$AB = BE + AE$

$= (30 + x)$ 

$AC = AF + FC$

$= (7 + x)$ 

$BC = BD + DC$

$= 30 + 7$

$= 37\ cm$

In right angled triangle $ABC$,

By Pythagoras theorem,

$BC^2 = AB^2 + AC^2$ 

$(37)^2 = (30 + x)^2 + (7 + x)^2$

$1369 = 900 + 60x + x^2 + 49 + 14x + x^2$

$2x^2 + 74x + 949 - 1369 = 0$

$2x^2+ 74x - 420 = 0$ 

$x^2 + 37x - 210 = 0$

$x^2 + 42x - 5x - 210 = 0$ 

$x (x + 42) - 5 (x + 42) = 0$

$(x - 5) (x + 42) = 0$

$(x - 5) = 0$ or $(x + 42) = 0$ 

$x = 5$ or $x = - 42$ 

$x = 5$    (Since x cannot be negative)

This implies,

$AF = 5\ cm$. 

The length of $AF$ is $5\ cm$.

Tutorialspoint

Simply Easy Learning

Updated on: 10-Oct-2022

28 Views

Kickstart Your Career

Get certified by completing the course

Get Started