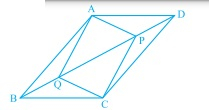
In parallelogram $ \mathrm{ABCD} $, two points $ \mathrm{P} $ and $ \mathrm{Q} $ are taken on diagonal $ \mathrm{BD} $ such that $ \mathrm{DP}=\mathrm{BQ} $ (see below figure). Show that:
(i) $ \triangle \mathrm{APD} \equiv \triangle \mathrm{CQB} $
(ii) $ \mathrm{AP}=\mathrm{CQ} $
(iii) $ \triangle \mathrm{AQB} \equiv \triangle \mathrm{CPD} $
(iv) $ \mathrm{AQ}=\mathrm{CP} $
(v) $ \mathrm{APCQ} $ is a parallelogram
"

Given:

In parallelogram \( \mathrm{ABCD} \), two points \( \mathrm{P} \) and \( \mathrm{Q} \) are taken on diagonal \( \mathrm{BD} \) such that \( \mathrm{DP}=\mathrm{BQ} \)

To do :

We have to show that

(i) \( \triangle \mathrm{APD} \equiv \triangle \mathrm{CQB} \)

(ii) \( \mathrm{AP}=\mathrm{CQ} \)

(iii) \( \triangle \mathrm{AQB} \equiv \triangle \mathrm{CPD} \)

(iv) \( \mathrm{AQ}=\mathrm{CP} \)

(v) \( \mathrm{APCQ} \) is a parallelogram

Solution :  

(i) In $\triangle APD$ and $\triangle CQB$,

$DP = BQ$         (Given)

$\angle ADP = \angle CBQ$            (Alternate interior angles are equal)

$AD = BC$     (Opposite sides of a parallelogram are equal)

Therefore, by SAS congruency,

$\triangle APD \cong \triangle CQB$

(ii) $\triangle APD \cong \triangle CQB$

This implies,

$AP = CQ$   (CPCT)

(iii) In $\triangle AQB$ and $\triangle DPC$,

$BQ = DP$           (Given)

$\angle ABQ = \angle CDP$         (Alternate interior angles are equal)

$AB = CD$         (Opposite sides of a parallelogram are equal)

Therefore, by SAS congruency,

$\triangle AQB \cong \triangle CPD$

(iv) $\triangle AQB \cong \triangle CPD$

This implies,

$AQ = CP$                (CPCT)

(v) $AP = CQ$

$AQ = CP$

This implies,

In quadrilateral $APCQ$ opposite sides are equal and opposite angles are equal.

Therefore, $APCQ$ is a parallelogram.

Tutorialspoint

Simply Easy Learning

Updated on: 10-Oct-2022

18 Views

Kickstart Your Career

Get certified by completing the course

Get Started