In ∆ABC and ∆PQR, AB = PQ, BC = QR and CB and RQ are extended to X and Y respectively and ∠ABX = ∠PQY. Prove that ∆ABC ≅ ∆PQR.
"

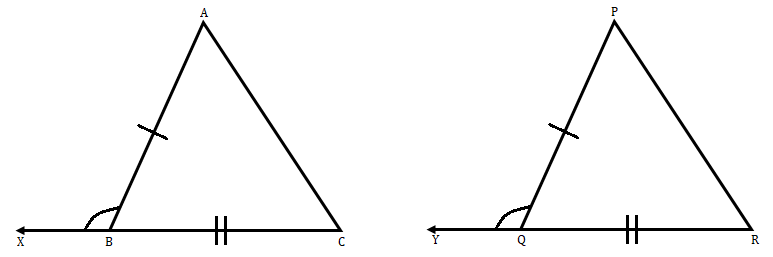Given: 

AB = PQ, BC = QR

CB and RQ are extended to X and Y respectively

∠ABX = ∠PQY

To prove: Here we have to prove that ∆ABC ≅ ∆PQR.

Solution:

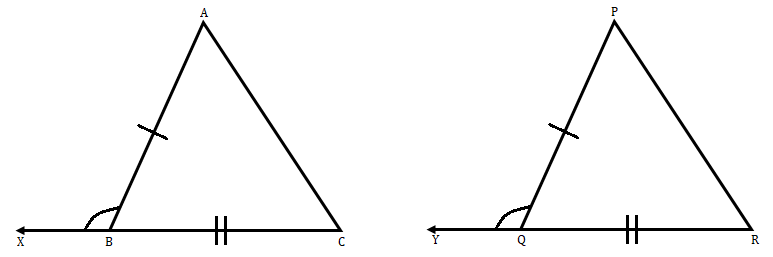
∠ABC $+$ ∠ABX = 180   (Straight angle)   ....(i)

∠PQR $+$ ∠PQY = 180   (Straight angle)   ....(ii)

From (i) and (ii):

∠ABC $+$ ∠ABX = ∠PQR $+$ ∠PQY

Given that, ∠ABX = ∠PQY

∠ABC = ∠PQR       ....(iii)                      

Now, in ∆ABC and ∆PQR:

AB = PQ                  (Given)

∠ABC = ∠PQR        (from eq. iii) 

BC = QR                  (Given)

So, ∆ABC ≅ ∆PQR by SAS criteria.