Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
If ∠B and ∠Q are acute angles such that $Sin B = Sin Q$, then prove that $∠B = ∠Q$.
Given:
$Sin B = Sin Q$
To do :
We have to prove that $∠ B = ∠ Q$.
Solution :
Consider two right triangles ABC and PQR where $sin B = sin Q$.
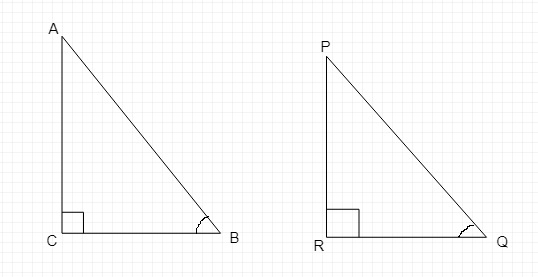
From the figures,
$Sin B = \frac{AC}{AB}$
and $Sin Q = \frac{PR}{PQ}$
This implies,
$\frac{AC}{AB} = \frac{PR}{PQ}$
$\frac{AC}{PR} = \frac{AB}{PQ}$
Let$\frac{AC}{PR} = \frac{AB}{PQ} = k$
$AC = k(PR)$ and $AB = k(PQ)$
In triangles, ABC and PQR, using the Pythagoras theorem,
$AC^2 + BC^2 = AB^2$ and $QR^2+PR^2=PQ^2$
$BC = \sqrt{(AB^2-AC^2)}$ and $QR = \sqrt{(PQ^2-PR^2)}$
$\frac{BC}{QR} = \frac{ \sqrt{(AB^2-AC^2)}}{\sqrt{(PQ^2-PR^2)}}$
$\frac{BC}{QR} = \frac{ \sqrt{(kPQ)^2-(kPR^2)}}{\sqrt{(PQ^2-PR^2)}}$
$\frac{BC}{QR} = \frac{ \sqrt{k^2 (PQ^2-PR^2)}}{\sqrt{(PQ^2-PR^2)}}$
$\frac{BC}{QR} = \frac{k \sqrt{(PQ^2-PR^2)}}{\sqrt{(PQ^2-PR^2)}}$
$\frac{BC}{QR} = k$
$\frac{AC}{PR} = \frac{AB}{PQ} = k$ and $\frac{BC}{QR} = k$
$\frac{AC}{PR} = \frac{AB}{PQ} = \frac{BC}{QR} $
In triangles ABC and PQR,
$\frac{AC}{PR} = \frac{AB}{PQ} = \frac{BC}{QR} $
Therefore,
$∆ ACB ~ ∆ PRQ$
This implies,
$∠ B = ∠ Q$.
Hence proved.
102 Views
