Given $ \overline{\mathrm{AB}} $ of length $ 7.3 \mathrm{~cm} $ and $ \overline{\mathrm{CD}} $ of length $ 3.4 \mathrm{~cm} $, construct a line segment $ \overline{X Y} $ such that the length of $ \overline{X Y} $ is equal to the difference between the lengths of $ \overline{\mathrm{AB}} $ and $ \overline{\mathrm{CD}} $. Verify by measurement.
Given:
Given $\overline{AB}$ of length $7.3\ cm$ and $\overline{CD}$ of length $3.4\ cm$.
To do:
We have to construct a line segment $\overline{XY}$ whose length is equal to the difference between the lengths of $\overline{AB}$ and $\overline{CD}$ and verify it by measurement.
Solution:
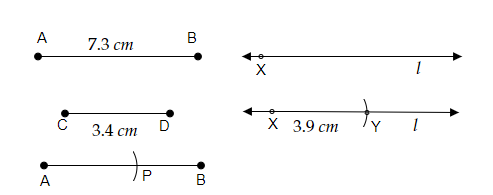
Steps of construction:
(i) First let us take compasses and by pointing the pointer of the compasses at point C of the line segment CD let us take the length of the line segment CD with compasses.
(ii) Now, by placing the pointer of the compasses on point A of the line segment AB let us draw an arc and point it as P.
(iii) let us draw the line 'l' and mark a point X on it.
(iv) let us adjust the compasses up to the length of PB, by pointing the pointer of compasses on point X let us draw an arc on the line 'l' and point it as point Y.
(v) Therefore, The line segment $\overline{XY}$ is formed.
(vi) The difference between the lengths of $\overline{AB}$ and $\overline{CD}$ is $7.3\ cm - 3.4\ cm = 3.9\ cm$.
(vii) We can verify the measurement by placing the ruler's starting point on point X to point Y measures $3.9\ cm$.
Related Articles
- Given some line segment \( \overline{\mathrm{AB}} \), whose length you do not know, construct \( \overline{\mathrm{PQ}} \) such that the length of \( \overline{\mathrm{PQ}} \) is twice that of \( \overline{\mathrm{AB}} \).
- Draw \( \overline{\mathrm{AB}} \) of length \( 7.3 \mathrm{~cm} \) and find its axis of symmetry.
- Draw any line segment \( \overline{\mathrm{PQ}} \). Without measuring \( \overline{\mathrm{PQ}} \), construct a copy of \( \overline{\mathrm{PQ}} \).
- Let \( \overline{\mathrm{PQ}} \) be the perpendicular to the line segment \( \overline{\mathrm{XY}} \). Let \( \overline{\mathrm{PQ}} \) and \( \overline{\mathrm{XY}} \) intersect in the point \( \mathrm{A} \). What is the measure of \( \angle \mathrm{PAY} \) ?
- Draw a circle with centre \( \mathrm{C} \) and radius \( 3.4 \mathrm{~cm} \). Draw any chord \( \overline{\mathrm{AB}} \). Construct the perpendicular bisector of \( \overline{\mathrm{AB}} \) and examine if it passes through \( \mathrm{C} \).
- State which of the following are triangles.\( \overline{A B}=7 \mathrm{~cm}, \overline{B C}=8 \mathrm{~cm}, \quad \overline{A C}=7 \mathrm{~cm} \)
- If \( \mathrm{B} \) is the mid point of \( \overline{\mathrm{AC}} \) and \( \mathrm{C} \) is the mid point of \( \overline{\mathrm{BD}} \), where \( \mathrm{A}, \mathrm{B}, \mathrm{C}, \mathrm{D} \) lie on a straight line, say why \( \mathrm{AB}=\mathrm{CD} \)?
- Draw any line segment \( \overline{\mathrm{AB}} \). Mark any point \( \mathrm{M} \) on it. Through \( \mathrm{M} \), draw a perpendicular to \( \overline{\mathrm{AB}} \). (use ruler and compasses)
- With \( \overline{\mathrm{PQ}} \) of length \( 6.1 \mathrm{~cm} \) as diameter, draw a circle.
- Draw \( \overline{A B} \) of length \( 7.3 \mathrm{~cm} \) and find its axis of symmetry.
- Draw a rough figure and label suitably in each of the following cases:(a) Point \( P \) lies on \( \overline{\mathrm{AB}} \).(b) \( \overline{\mathrm{XY}} \) and \( \overline{\mathrm{PQ}} \) intersect at \( \mathrm{M} \).(c) Line \( l \) contains \( \bar{E} \) and \( \bar{F} \) but not \( \bar{D} \).(d) \( \overline{\mathrm{OP}} \) and \( \overline{\mathrm{OQ}} \) meet at \( O \).
- Draw the perpendicular bisector of \( \overline{X Y} \) whose length is \( 10.3 \mathrm{~cm} \).(a) Take any point \( \mathrm{P} \) on the bisector drawn. Examine whether \( \mathrm{PX}=\mathrm{PY} \).(b) If \( \mathrm{M} \) is the mid point of \( \overline{\mathrm{XY}} \), what can you say about the lengths \( \mathrm{MX} \) and \( \mathrm{XY} \) ?
- Verify, whether \( \mathrm{D} \) is the mid point of \( \overline{\mathrm{AG}} \)."
- Draw a line $l$ and a point \( \mathrm{X} \) on it. Through \( \mathrm{X} \), draw a line segment \( \overline{\mathrm{XY}} \) perpendicular to $1$. Now draw a perpendicular to \( \overline{X Y} \) at Y. (use ruler and compasses)
Kickstart Your Career
Get certified by completing the course
Get Started
Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies