Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
From each of the two opposite corners of a square of side $ 8 \mathrm{~cm} $, a quadrant of a circle of radius $ 1.4 \mathrm{~cm} $ is cut. Another circle of radius $ 4.2 \mathrm{~cm} $ is also cut from the centre as shown in the figure. Find the area of the remaining (shaded) portion of the square. $ ( $ Use $ \pi=22 / 7) $.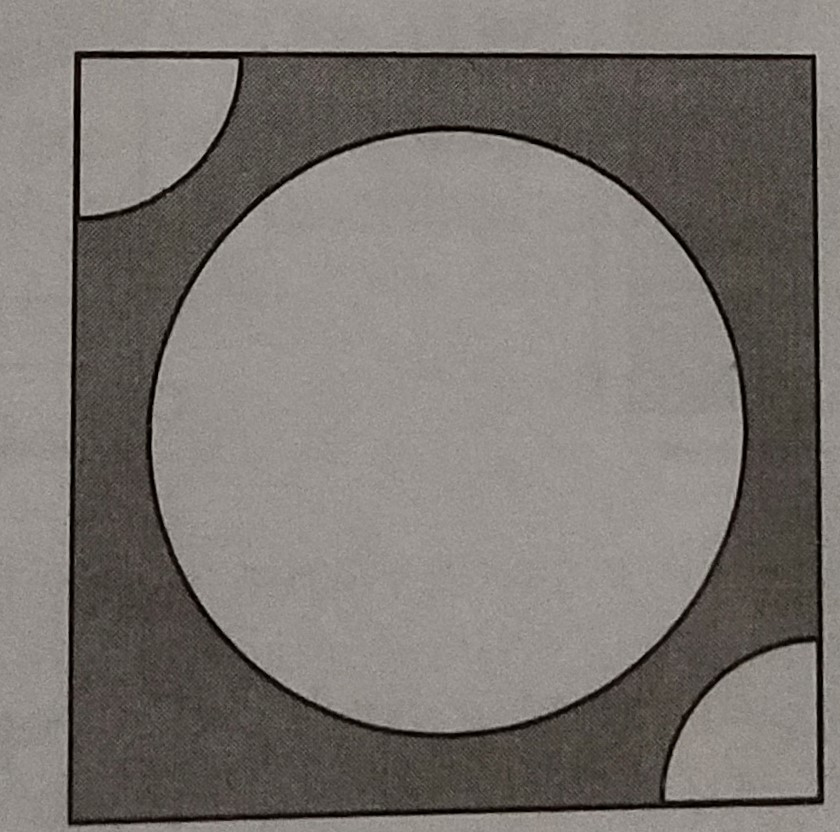
"
Given:
From each of the two opposite corners of a square of side \( 8 \mathrm{~cm} \), a quadrant of a circle of radius \( 1.4 \mathrm{~cm} \) is cut. Another circle of radius \( 4.2 \mathrm{~cm} \) is also cut from the centre as shown in the figure.
To do:
We have to find the area of the remaining (shaded) portion of the square.
Solution:
Length of the side of the square $ABCD = 8\ cm$
This implies,
Area of the square $=(8)^{2}$
$=64 \mathrm{~cm}^{2}$
Radius of each quadrant $=1.4 \mathrm{~cm}$
This implies,
Area of two quadrants $=2 \times \frac{1}{4} \pi r^{2}$
$=\frac{1}{2} \times \frac{22}{7}(1.4)^{2} \mathrm{~cm}^{2}$
$=\frac{1}{2} \times \frac{22}{7} \times 1.4 \times 1.4$
$=3.08 \mathrm{~cm}^{2}$
Diameter of the circle $=4.2 \mathrm{~cm}$
This implies,
Radius of the circle $=\frac{4.2}{2}$
$=2.1 \mathrm{~cm}$
Area of the circle $=\pi r^{2}$
$=\frac{22}{7}(2.1)^{2} \mathrm{~cm}^{2}$
$=\frac{22}{7} \times 2.1 \times 2.1$
$=13.86 \mathrm{~cm}^{2}$
Therefore,
Area of the shaded portion $=64-(3.08+13.86) \mathrm{cm}^{2}$
$=64-16.94$
$=47.06 \mathrm{~cm}^{2}$
The area of the remaining (shaded) portion of the square is $47.06\ cm^2$.
25 Views
