Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
Construct an angle of $ 90^{\circ} $ at the initial point of a given ray and justify the construction.
To do:
We have to construct an angle of $90^o$ at the initial point of a given ray and justify the construction.
Solution:
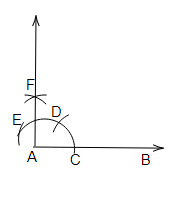
Steps of construction:
(a) Draw a ray $AB$.
(b) With centre $A$ and a suitable radius, draw an arc such that it cuts $AB$ at $C$.
(c) With centre $C$ and the same radius taken above cut the above arc at $D$.
(d) With centre $D$ and the same radius taken above cut the above arc at $E$.
(c) With $D$ and $E$ as centers and radius more than $\frac{1}{2}DE$ draw two arcs meeting each other at $F$.
(d) Join $AF$ and produce it to form ray $AF$.
Therefore,
$\angle BAF = 90^o$.
Justification:
To justify $\angle BAF=90^o$
Let us draw an imaginary line from $A$ to $D$ and $O$ to $E$.
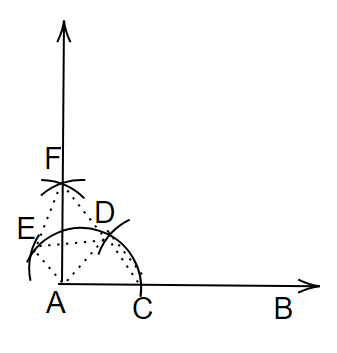
We have,
$AC=CD=AD$
Therefore,
$ACD$ is an equilateral triangle
This implies,
$\angle CAD=60^o$
In a similar way, we get,
$AE=DE=AD$
Therefore,
$ADE$ is an equilateral triangle
This implies,
$\angle EAD=60^o$
By SSS congruence rule we get,
$\triangle ACD \cong \triangle ADE$
From C.P.C.T We get,
$\angle CAD=\angle EAD$
Therefore,
$\angle DAF=\frac{1}{2}EAD=\frac{1}{2}(60^o)=30^o$
This implies,
$\angle DAF=30^o$
$\angle BAF=\angle BAD+\angle DAF$
$=60^o+30^o$
$=90^o$
Therefore, justified.
28 Views
