- Trending Categories
Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
A solid metallic right circular cone 20 cm high and whose vertical angle is $60^{o}$, is cut into two parts at the middle of its height by a plane parallel to it base.If the frustum so obtained drawn into a wire of diameter $\frac{1}{12}$cm, find the length of the wire.
Given: A solid metallic right circular cone 20 cm high and whose vertical angle is $60^{o}$, is cut into two parts at the middle of its height by a plane parallel to it base. If the frustum so obtained drawn into a wire of diameter $\frac{1}{12}$cm.
To do: To find the length of the wire.
Solution:
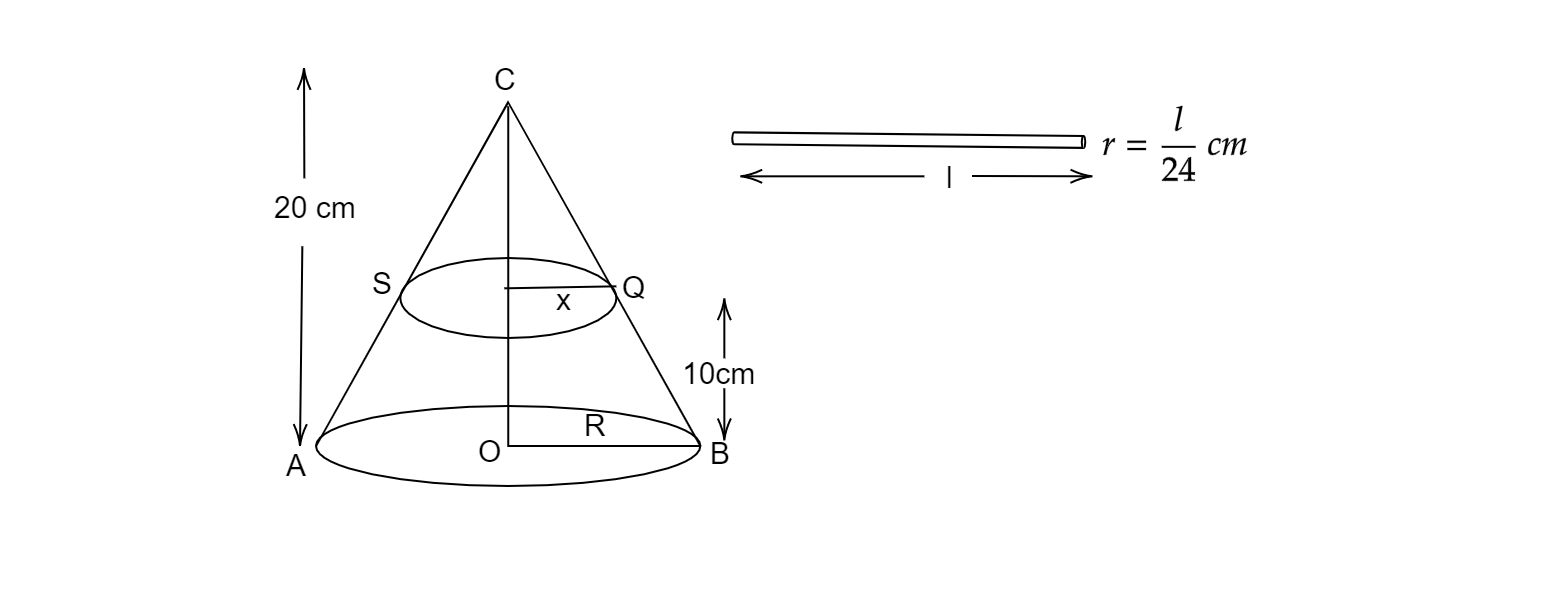
Let ACB be the cone whose vertical angle $\angle ACB = 60^{o} $.
Let $R$ and $x$ be the radii of the lower and upper end of the frustum.
Here, height of the cone, $OC = 20 cm=H$
Height $CP = h = 10\ cm $
Let us consider P as the mid-Point of OC
Cutting the cone into two parts through P.
OP =$\frac{20}{2}= 10\ cm$
Also,$\angle ACO$ and $\angle OCB =$\frac{1}{2} \times 60^{o} =30^{o} $
After cutting cone CQS from cone CBA, the remaining solid obtained is a frustum.
Now, in triangle CPQ
$tan30^{o}=\frac{x}{10}$
$\frac{1}{\sqrt{3}} =\frac{x}{10}$
$\Rightarrow x=\frac{10}{\sqrt{3}}\ cm$
In triangle COB
$tan30^{o}=\frac{R}{20}$
$\Rightarrow \frac{1}{\sqrt{3}} =\frac{R}{20}$
$\Rightarrow R=\frac{20}{\sqrt{3}}$
Volume of the frustum, $V=\frac{1}{3} \pi \left( R^{2} H-x^{2} h\right)$
$\Rightarrow V=\frac{1}{3} \pi \left(\left(\frac{20}{\sqrt{3}}\right)^{2} .20-\left(\frac{10}{\sqrt{3}}\right)^{2} .10\right)$
$\Rightarrow V=\frac{1}{3} \pi \left(\frac{400\times 20}{3} -\frac{100\times 10}{3}\right)$
$\Rightarrow V=\frac{1}{3} \pi \left(\frac{8000-1000}{3}\right)$
$\Rightarrow V=\frac{7000}{9} \pi \ cm^{3}$
Let us assume the length of the wire l.
Given diameter of the wire obtained from the frustum$=\frac{1}{12}\ cm$
Radius of the wire, $r=\frac{1}{2} \times \frac{1}{12} =\frac{1}{24}\ cm$
Volume of the wire$=\pi r^{2} l$
$=\pi \left(\frac{1}{24}\right)^{2} l$
$=\frac{\pi l}{576} cm^{3}$
The volumes of the frustum and the wire formed are equal,
$\frac{7000}{9} \pi =\frac{\pi l}{576}$
$\Rightarrow \frac{7000}{9} =\frac{l}{576}$
$\Rightarrow l=\frac{7000\times 576}{9}$
$\Rightarrow l=448000\ cm$
$\Rightarrow l=4480\ cm$
Therefore, length of the wire is 480 cm.
Updated on: 10-Oct-2022
33 Views
Advertisements