$ A $ is a point at a distance $ 13 \mathrm{~cm} $ from the centre $ O $ of a circle of radius $ 5 \mathrm{~cm} $. $ A P $ and $ A Q $ are the tangents to the circle at $ P $ and $ Q $. If a tangent $ B C $ is drawn at a point $ R $ lying on the minor arc $ P Q $ to intersect $ A P $ at $ B $ and $ A Q $ at $ C $, find the perimeter of the $ \triangle A B C $.

Given:

\( A \) is a point at a distance \( 13 \mathrm{~cm} \) from the centre \( O \) of a circle of radius \( 5 \mathrm{~cm} \). \( A P \) and \( A Q \) are the tangents to the circle at \( P \) and \( Q \).

A tangent \( B C \) is drawn at a point \( R \) lying on the minor arc \( P Q \) to intersect \( A P \) at \( B \) and \( A Q \) at \( C \).

To do:

We have to find the perimeter of the \( \triangle A B C \).

Solution:

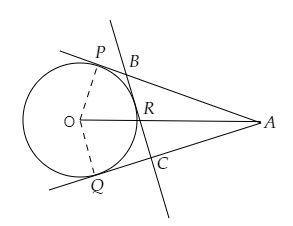
$\angle OPA = 90^o$    (Tangent at a point on a circle is perpendicular to the radius through the point of contact)

In $\triangle OPA$,

$OA^2 = OP^2 + PA^2$    (By Pythagoras theorem)

$(13)^2 = 5^2 + PA^2$

$PA^2 = 169-25$

$=144$

$=(12)^2$

$\Rightarrow PA = 12\ cm$

Perimeter of $\triangle ABC = AB + BC + CA$

$= (AB + BR) + (RC + CA)$

$= AB + BP + CQ + CA$   (since $BR = BP$ and $RC = CQ$, Tangents from internal point to a circle are equal)

$= AP + AQ$ 

$= 2AP$        (Tangents from internal point to a circle are equal)

$= 2 \times 12$

$= 24\ cm$

The perimeter of $\triangle ABC$ is $24\ cm$.