A circus artist is climbing a $ 20 \mathrm{~m} $ long rope, which is tightly stretched and tied from the top of a vertical pole to the ground. Find the height of the pole, if the angle made by the rope with the ground level is $ 30^{\circ} $.
Given:
Length of the rope$=20\ m$.
The angle made by the rope with the ground level is \( 30^{\circ} \).
To do:
We have to find the height of the pole.
Solution:
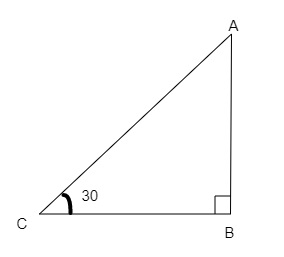
Let AB be the height of the pole and AC the length of the rope.
Angle made by the rope with the ground $\angle BCA=30^o$
Therefore,
$sin30^o=\frac{AB}{AC}$
$\frac{1}{2}=\frac{AB}{20}$
$AB=\frac{20}{2}$
$AB=10\ m$
The height of the pole is $10\ m$.
Related Articles
- A circus artist is climbing a $20\ m$ long rope, which is tightly stretched and tied from the top of a vertical pole to the ground. Find the height of the pole, if the angle made by the rope with the ground level is $30^o$ (see figure)."
- An electric pole is \( 10 \mathrm{~m} \) high. A steel wire tied to top of the pole is affixed at a point on the ground to keep the pole up right. If the wire makes an angle of \( 45^{\circ} \) with the horizontal through the foot of the pole, find the length of the wire.
- The horizontal distance between two poles is \( 15 \mathrm{~m} \). The angle of depression of the top of the first pole as seen from the top of the second pole is \( 30^{\circ} \). If the height of the second pole is \( 24 \mathrm{~m} \), find the height of the first pole. \( (\sqrt{3}=1.732) \quad \)
- A tower stands vertically on the ground. From a point on the ground, \( 20 \mathrm{~m} \) away from the foot of the tower, the angle of elevation of the top of the tower is \( 60^{\circ} \). What is the height of the tower?
- A calf is tied with a rope of length \( 6 \mathrm{~m} \) at the corner of a square grassy lawn of side \( 20 \mathrm{~m} \). If the length of the rope is increased by \( 5.5 \mathrm{~m} \), find the increase in area of the grassy lawn in which the calf can graze.
- The angle of elevation of the top of a tower from a point on the ground, which is $30\ m$ away from the foot of the tower is $30^o$. Find the height of the tower.
- A pole \( 6 \mathrm{~m} \) high casts a shadow \( 2 \sqrt{3} \mathrm{~m} \) long on the ground, then the Sun's elevation is(A) \( 60^{\circ} \)(B) \( 45^{\circ} \)(C) \( 30^{\circ} \)(D) \( 90^{\circ} \)
- A vertical pole of length 6 m casts a shadow 4 m long on the ground and at the same time a tower casts a shadow \( 28 \mathrm{~m} \) long. Fin the height of the tower.
- From the top of a \( 50 \mathrm{~m} \) high tower, the angles of depression of the top and bottom of a pole are observed to be \( 45^{\circ} \) and \( 60^{\circ} \) respectively. Find the height of the pole..
- A vertical pole of length 6 m casts a shadow 4 m long on the ground and at the same time a tower casts a shadow 28 m long. Find the height of the tower.
- A flag pole \( 18 \mathrm{~m} \) high casts a shadow \( 9.6 \mathrm{~m} \) long. Find the distance of the top of the pole from the far end of the shadow.
- A street light bulb is fixed on a pole \( 6 \mathrm{~m} \) above the level of the street. If a woman of height \( 1.5 \mathrm{~m} \) casts a shadow of \( 3 \mathrm{~m} \), find how far she is away from the base of the pole.
- On a horizontal plane there is a vertical tower with a flag pole on the top of the tower. At a point 9 metres away from the foot of the tower the angle of elevation of the top and bottom of the flag pole are \( 60^{\circ} \) and \( 30^{\circ} \) respectively. Find the height of the tower and the flag pole mounted on it.
- The horizantol distance between two poles is 15 cm. The angle of depression of the top of first pole as seen from the top of second pole is 30$^{o}$. If the height of the second pole is 24 cm, find the height of the first pole. [use $\pi =\frac{22}{7}$] .
- A tree breaks due to storm and the broken part bends so that the top of the tree touches the ground making an angle of \( 30^{\circ} \) with the ground. The distance between the foot of the tree to the point where the top touches the ground is \( 8 \mathrm{~m} \). Find the height of the tree.
Kickstart Your Career
Get certified by completing the course
Get Started
Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies