Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
A chord of a circle of radius 14cm subtends an angle of 120° at the centre. Find the area of the corresponding minor segment of the circle. $\left( Use\ \pi =\frac{22}{7} \ and\ \sqrt{3} =1.73\right) \ $
Given: A circle of radius 14 cm, and a chord subtending an angle $120^{o}$.
To do: To find out the area of the minor segment of the circle.
Solution: Let us say there is a circle with centre O, and AB is the given chord as shown in the fig.
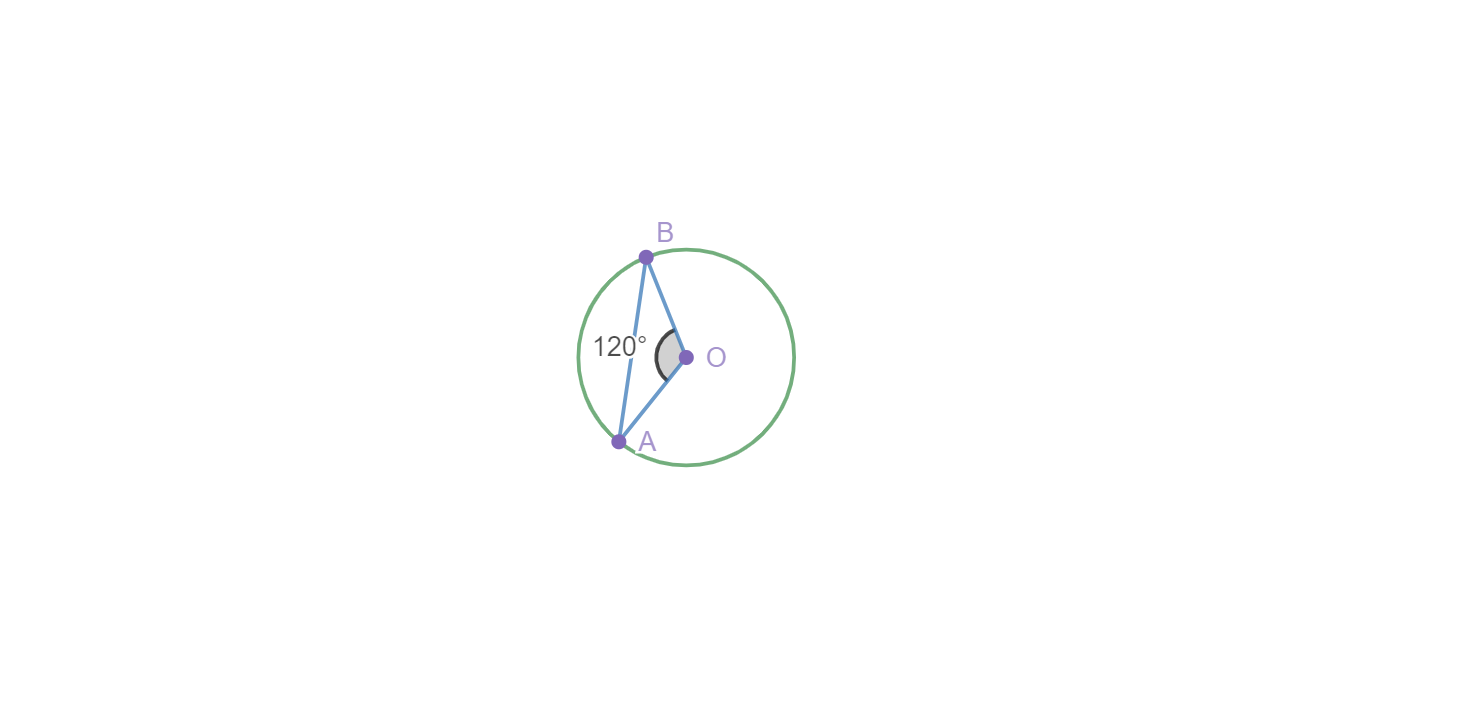
Here given radius of the given circle, $r=14\ cm$
Angle subtended by the chord AB, $\angle AOB=120^{o}$.
Here first we’ll find out area of the sector $AOB$ and then area of the triangle $\vartriangle AOB$.
Area of the sector $AOB=\frac{\theta }{360^{o}} \pi r^{2}$
Here $\theta=120^{o}$ and$\ r=14\ cm$
$=\frac{120^{o}}{360^{o}} \times \frac{22}{7} \times ( 14)^{2}$
$=\frac{1}{3} \times \frac{22}{7} \times 14\times 14$
$=\frac{616}{3} \ cm^{2}$
Area of the sector $AOB=205.33\ cm^{2}$
Area of the $\vartriangle ABC=\frac{1}{2} \times OA\times OB\times sin120^{o}$
Here $OA=OB=$radius of the given circle=14\ cm$
And we know $sin120^{o} =\frac{\sqrt{3}}{2}$, On submitting these values in the formula,
Area of $\vartriangle ABC=\frac{1}{2} \times 14\times 14\times \frac{\sqrt{3}}{2}$
$=49\sqrt{3}$
$=49\times 1.73$
$=84.77\ cm^{2}$
Area of the minor segment of the circle $=$area of the sector AOB$-$area of $\vartriangle AOB$
$\ =205.33-84.77$
$=120.56\ cm^{2}$
Thus the area of the minor segment is $120.56\ cm^{3}$.
Updated on: 10-Oct-2022
2K+ Views
Advertisements