Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
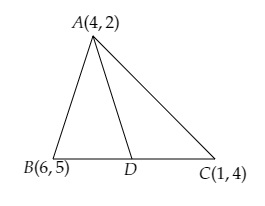
Let $A(4, 2), B(6,5)$ and $C(1, 4)$ be the vertices of $∆ABC$.
(i) The median from $A$ meets $BC$ at $D$. Find the coordinates of the point $D$.
(ii) Find the coordinates of the point $P$ on $AD$, such that $AP : PD = 2 : 1$.
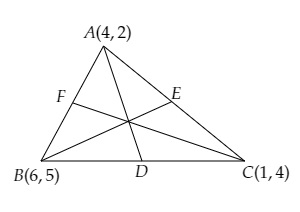
(iii) Find the coordinates of points $Q$ and $R$ on medians $BE$ and $CF$ respectively, such that $BQ : QE = 2 : 1$ and $CR : RF = 2 : 1$.
(iv) What do you observe?
[Note: The point which is common to all the three medians is called centroid and this point divides each median in the ratio 2: 1]
(v) If $A(x_1, y_1), B(x_2, y_2)$ and $C(x_3, y_3)$ are the vertices of $∆ABC$, find the coordinates of the centroid of the triangles.
To do:
We have to find:
(i) The coordinates of point D.
(ii) Find the coordinates of the point $P$ on $AD$, such that $AP : PD = 2 : 1$.
(iii) Find the coordinates of points $Q$ and $R$ on medians $BE$ and $CF$ respectively, such that $BQ : QE = 2 : 1$ and $CR : RF = 2 : 1$.
(iv) What do we observe
[Note: The point which is common to all the three medians is called centroid and this point divides each median in the ratio of 2: 1]
(v) If $A(x_1, y_1), B(x_2, y_2)$ and $C(x_3, y_3)$ are the vertices of $∆ABC$, find the coordinates of the centroid of the triangles.
Solution:
(i) $D$ is the mid-point of BC.
This implies,
Using the mid-point formula, we get,
Coordinates of $D=(\frac{6+1}{2}, \frac{5+4}{2})$
$=(\frac{7}{2},\frac{9}{2})$
The coordinates of the point $D$ are $(\frac{7}{2},\frac{9}{2})$ .
(ii) $D$ is the mid-point of BC.
This implies,
Using the mid-point formula, we get,
Coordinates of $D=(\frac{6+1}{2}, \frac{5+4}{2})$
$=(\frac{7}{2},\frac{9}{2})$
$AP : PD = 2 : 1$.
Using the section formula, we get,
$(x,y)=(\frac{m x_{2}+n x_{1}}{m+n}, \frac{m y_{2}+n y_{1}}{m+n})$
The coordinates of $\mathrm{P}$ are $(\frac{2 \times \frac{7}{2}+1 \times 4}{1+2}, \frac{2 \times \frac{9}{2}+1 \times 2}{1+2})$
$=(\frac{7+4}{3}, \frac{9+2}{3})$
$=(\frac{11}{3}, \frac{11}{3})$
The coordinates of $\mathrm{P}$ are $(\frac{11}{3}, \frac{11}{3})$.
(iii) $D$ is the mid-point of BC.
This implies,
Using the mid-point formula, we get,
Coordinates of $D=(\frac{6+1}{2}, \frac{5+4}{2})$
$=(\frac{7}{2},\frac{9}{2})$
Similarly,
Coordinates of $E=(\frac{4+1}{2}, \frac{2+4}{2})$
$=(\frac{5}{2},3)$
Coordinates of $F=(\frac{4+6}{2}, \frac{2+5}{2})$
$=(5,\frac{7}{2})$
$AP : PD = 2 : 1$.
Using the section formula, we get,
$(x,y)=(\frac{m x_{2}+n x_{1}}{m+n}, \frac{m y_{2}+n y_{1}}{m+n})$
The coordinates of $\mathrm{P}$ are $(\frac{2 \times \frac{7}{2}+1 \times 4}{1+2}, \frac{2 \times \frac{9}{2}+1 \times 2}{1+2})$
$=(\frac{7+4}{3}, \frac{9+2}{3})$
$=(\frac{11}{3}, \frac{11}{3})$
$BQ : QE = 2 : 1$
The coordinates of $\mathrm{Q}$ are $(\frac{2 \times \frac{5}{2}+1 \times 6}{1+2}, \frac{2 \times 3+1 \times 5}{1+2})$
$=(\frac{5+6}{3}, \frac{6+5}{3})$
$=(\frac{11}{3}, \frac{11}{3})$
$CR : RF = 2 : 1$
The coordinates of $\mathrm{R}$ are $(\frac{2 \times 5+1 \times 1}{1+2}, \frac{2 \times \frac{7}{2}+1 \times 4}{1+2})$
$=(\frac{10+1}{3}, \frac{7+4}{3})$
$=(\frac{11}{3}, \frac{11}{3})$
We observe that the coordinates of $P, Q$ and $R$ are the same. $P, Q$ and $R$ coincide with each other.
Medians of the sides of a triangle pass through the same point which is called the centroid of the triangle.
Therefore, $(\frac{11}{3},\frac{11}{3})$ is the centroid of the triangle ABC.
The coordinates of $\mathrm{Q}$ and $\mathrm{R}$ are $(\frac{11}{3}, \frac{11}{3})$.
(iv) Since, the coordinates of $\mathrm{P}, \mathrm{Q}$ and $\mathrm{R}$ are $(\frac{11}{3}, \frac{11}{3}, \frac{11}{3})$ this shows that medians of $P, Q$ and $R$ intersect each other at centroid of the $\triangle {ABC}$.
(v)
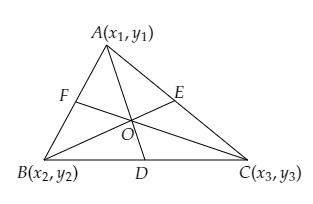
We know that,
Coordinates of the centroid of a triangle are $(\frac{Sum\ of\ abscissa}{3}, \frac{Sum\ of\ ordinates}{3})$
Therefore,
The coordinates of the centroid of triangle ABC are,
$(\frac{x_1+x_2+x_3}{3}, \frac{y_1+y_2+y_3}{3})$
The coordinates of the centroid of triangle ABC are $(\frac{x_1+x_2+x_3}{3}, \frac{y_1+y_2+y_3}{3})$.
Similarly,
The coordinates of the centroid of triangle ABC whose vertices are $A(4, 2), B(6,5)$ and $C(1, 4)$ are
$(\frac{4+6+1}{3}, \frac{2+5+4}{3})=(\frac{11}{3}, \frac{11}{3})$
21 Views
