In figure below, if $ \mathrm{DE} \| \mathrm{BC} $, find the ratio of ar (ADE) and ar (DECB).
"

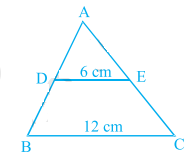Given:

\( \mathrm{DE} \| \mathrm{BC} \)

To do:

We have to find the ratio of ar (ADE) and ar (DECB).

Solution:

In $\triangle A B C$ and $\triangle A D E$,

$\angle A B C=\angle A D E$             (Corresponding angles)

$\angle A C B=\angle A E D$              (Corresponding angles)

$\angle A =\angle A$

Therefore, by AA similarity,

$\triangle A B C \sim \triangle A E D$

This implies,

$\frac{\operatorname{ar}(\triangle A D E)}{\operatorname{ar}(\triangle A B C)}=(\frac{DE}{BC})^2$

$=\frac{(6)^{2}}{(12)^{2}}$

$=(\frac{1}{2})^{2}$

$=\frac{1}{4}$

Let $ar (\triangle A D E)=k$

This implies,

$ar (\triangle A B C)=4 k$

$ar (D E C B)=\operatorname{ar}(A B C)-\operatorname{ar}(A D E)$

$=4 k-k$

$=3 k$

Therefore,

$\operatorname{ar}(A D E): \operatorname{ar}(D E C B)=k: 3 k$

$=1: 3$

The ratio of ar (ADE) and ar (DECB) is $1:3$.

Tutorialspoint

Simply Easy Learning

Updated on: 10-Oct-2022

195 Views

Kickstart Your Career

Get certified by completing the course

Get Started