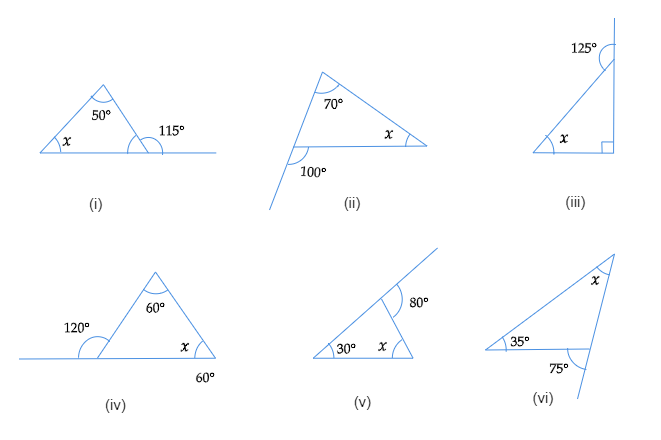
Find the value of the unknown interior angle x in the following figures:
"

To do:

We have to find the value of unknown interior angle $x$ in each case.

Solution: 

For convenience, we shall name all the triangles given in the diagram as $\triangle ABC$.

We know that,

An exterior angle of a triangle is equal to the sum of its interior opposite angles.

Therefore,

(i) $\angle A+x=\angle ACD$ 

$\Rightarrow 50^{\circ}+\ x=115^{\circ}$

$\Rightarrow x=115^{\circ}-50^{\circ}$

$\Rightarrow x=65^{\circ}$

(ii) $\angle A+x=\angle CBD$

$\Rightarrow 70^{\circ}+\ x=100^{\circ}$

$\Rightarrow x=100^{\circ}-\ 70^{\circ}$

$\Rightarrow x=30^{\circ}$

(iii) $\angle C+x=\angle BAD$

$\Rightarrow 90^{\circ}+x=125^{\circ}$

$\Rightarrow x=125^{\circ}-\ 90^{\circ}$

$\Rightarrow x=35^{\circ}$

(iv) $\angle A+x=\angle ABD$

$\Rightarrow 60^{\circ}+\ x=120^{\circ}$

$\Rightarrow x=120^{\circ}-60^{\circ}$

$\Rightarrow x = 60^{\circ}$

(v) $\angle B+x=\angle CAD$

$\Rightarrow 30^{\circ}+\ x=80^{\circ}$

$\Rightarrow x=80^{\circ}-\ 30^{\circ}$

$\Rightarrow x=50^{\circ}$

(vi) $\angle B+x=\angle BCD$

$\Rightarrow 35^{\circ}+\ x=75^{\circ}$

$\Rightarrow x=75^{\circ}-35^{\circ}$

$\Rightarrow x=40^{\circ}$

Tutorialspoint

Simply Easy Learning

Updated on: 10-Oct-2022

33 Views

Kickstart Your Career

Get certified by completing the course

Get Started