Construct $∆ABC$ with $BC = 7.5\ cm$, $AC = 5\ cm$ and $\angle C = 60^{\circ}$.
To do: To construct $∆ABC$ with $BC = 7.5\ cm$, $AC = 5\ cm$ and $m \angle C = 60^{\circ}$.
Steps of construction :
- Let us draw a line segment $BC$ with the length of $7.5\ cm$.
- From the point $C$, let us draw a ray $CX$ making an angle of $60^{\circ}$ with $BC$ such that $\angle ACB=60^{\circ}$.
- Assuming $C$ as the center, let us draw an arc of radius $5\ cm$ that cuts $CX$ at the point $A$.
- Now let us join $AB$ to get the required triangle.
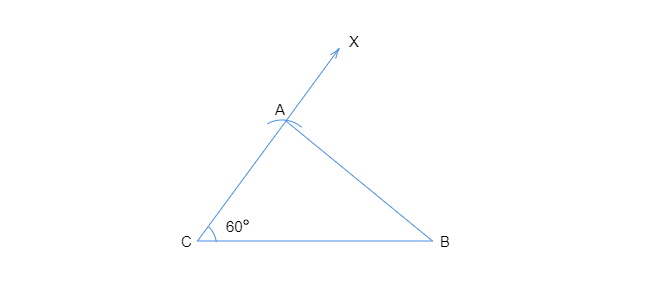
$\triangle ABC$ is the required triangle.
Hence constructed!
Related Articles
- Construct a $∆ABC$ in which $BC = 3.6\ cm, AB + AC = 4.8\ cm$ and $\angle B = 60^o$.
- Using ruler and compasses only, construct a $∆ABC$, given base $BC = 7\ cm, \angle ABC = 60^o$ and $AB + AC = 12\ cm$.
- Construct $∆ABC$ such that $AB=2.5\ cm$, $BC=6\ cm$ and $AC=6.5\ cm$. Measure $\angle B$.
- Construct $∆ABC$, given $m\angle A = 60^{\circ}$, $m\angle B = 30^{\circ}$ and $AB = 5.8\ cm$.
- Construct a $∆ABC$ in which $AB + AC = 5.6\ cm, BC = 4.5\ cm$ and $\angle B = 45^o$.
- ∆ABC ~ ∆LMN. In ∆ABC, AB = 5.5 cm, BC = 6 cm, CA = 4.5 cm. Construct ∆ABC and ∆LMN such that $\frac{BC}{MN} \ =\ \frac{5}{4}$.
- Construct $∆DEF$ such that $DE = 5\ cm$, $DF = 3\ cm$ and $\angle EDF = 90^{\circ}$.
- Construct $\vartriangle ABC$ in which $BC=7\ cm,\ \angle B=75^{o}$ and $AB+AC=12\ cm$.
- Construct a $\triangle ABC$ in which $BC = 3.4\ cm, AB - AC = 1.5\ cm$ and $\angle B = 45^o$.
- Construct a triangle \( \mathrm{ABC} \) in which \( \mathrm{BC}=7 \mathrm{~cm}, \angle \mathrm{B}=75^{\circ} \) and \( \mathrm{AB}+\mathrm{AC}=13 \mathrm{~cm} \).
- Construct a triangle \( \mathrm{ABC} \) in which \( \mathrm{BC}=8 \mathrm{~cm}, \angle \mathrm{B}=45^{\circ} \) and \( \mathrm{AB}-\mathrm{AC}=3.5 \mathrm{~cm} \).
- Construct an isosceles right-angled triangle $ABC$, where $m\angle ACB = 90^{\circ}$ and $AC = 6\ cm$.
- Tick the correct answer and justify : In $∆ABC, AB = 6\sqrt3\ cm, AC = 12\ cm$ and $BC = 6\ cm$. The angle B is:(a) $120^o$(b) $60^o$(c) $90^o$(d) $45^o$
- Construct $∆PQR$ if $PQ = 5\ cm$, $m\angle\ PQR = 105^{\circ}$ and $m\angle QRP = 40^{\circ}$. [Hint: Recall angle-sum property of a triangle.]
- Construct a triangle similar to \( \triangle A B C \) in which \( A B=4.6 \mathrm{~cm}, \mathrm{BC}=5.1 \mathrm{~cm}, \angle A=60^{\circ} \) with scale factor \( 4: 5 \).
Kickstart Your Career
Get certified by completing the course
Get Started
Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies