- Trending Categories
Data Structure
Networking
RDBMS
Operating System
Java
MS Excel
iOS
HTML
CSS
Android
Python
C Programming
C++
C#
MongoDB
MySQL
Javascript
PHP
Physics
Chemistry
Biology
Mathematics
English
Economics
Psychology
Social Studies
Fashion Studies
Legal Studies
- Selected Reading
- UPSC IAS Exams Notes
- Developer's Best Practices
- Questions and Answers
- Effective Resume Writing
- HR Interview Questions
- Computer Glossary
- Who is Who
An organisation selected 2400 families at random and surveyed them to determine a
relationship between income level and the number of vehicles in a family. The information gathered is listed in the table below:
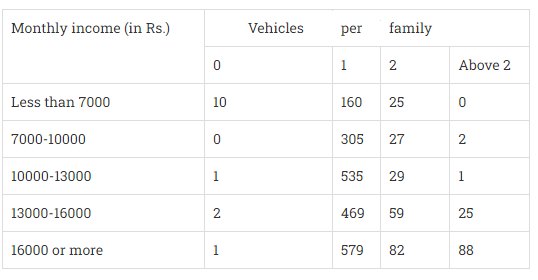
Suppose a family is chosen. Find the probability that the family chosen is
(i) earning $ Rs. 10000-13000 $ per month and owning exactly 2 vehicles.
(ii) earning $ Rs. 16000 $ or more per month and owning exactly 1 vehicle.
(iii) earning less than Rs. 7000 per month and does not own any vehicle.
(iv) earning $ Rs. 13000-16000 $ per month and owning more than 2 vehicles.
(v) owning not more than 1 vehicle."
Given:
An organisation selected 2400 families at random and surveyed them to determine a relationship between income level and the number of vehicles in a family.
A family is chosen.
To do:
We have to find the probability that the family chosen is
(i) earning \( Rs. 10000-13000 \) per month and owning exactly 2 vehicles.(ii) earning \( Rs. 16000 \) or more per month and owning exactly 1 vehicle.
(iii) earning less than Rs. 7000 per month and does not own any vehicle.
(iv) earning \( Rs. 13000-16000 \) per month and owning more than 2 vehicles.
(v) owning not more than 1 vehicle.
Solution:
(i) Total number of families $=2400$
Number of families earning Rs 10000-13000 per month and owning exactly 2 vehicles $=29$
We know that,
Probability of an event=$ \frac{Number \ of \ favourable \ outcomes}{Total \ number \ of \ outcomes}$
Therefore,
Probability that the family is earning Rs 10000-13000 per month and owning exactly 2 vehicles $=\frac{29}{2400}$
The probability the family is earning Rs 10000-13000 per month and owning exactly 2 vehicles is $\frac{29}{2400}$.
(ii) Total number of families $=2400$
Number of families earning Rs 16000 or more per month and owning exactly 1 vehicle $=579$
We know that,
Probability of an event=$ \frac{Number \ of \ favourable \ outcomes}{Total \ number \ of \ outcomes}$
Therefore,
Probability that the family is earning Rs 16000 or more per month and owning exactly 1 vehicle $=\frac{579}{2400}$
The probability the family is earning Rs 16000 or more per month and owning exactly 1 vehicle is $\frac{579}{2400}$.
(iii) Total number of families $=2400$
Number of families earning less than Rs 7000 per month and does not own any vehicle $=10$
We know that,
Probability of an event=$ \frac{Number \ of \ favourable \ outcomes}{Total \ number \ of \ outcomes}$
Therefore,
Probability that the family is earning less than Rs 7000 per month and does not own any vehicle $=\frac{10}{2400}$
$=\frac{1}{240}$
The probability the family is earning less than Rs 7000 per month and does not own any vehicle is $\frac{1}{240}$.
(iv) Total number of families $=2400$
Number of families earning Rs 13000-16000 per month and owning more than 2 vehicles $=25$
We know that,
Probability of an event=$ \frac{Number \ of \ favourable \ outcomes}{Total \ number \ of \ outcomes}$
Therefore,
Probability that the family is earning Rs 13000-16000 per month and owning more than 2 vehicles $=\frac{25}{2400}$
$=\frac{1}{96}$
The probability the family is earning Rs 13000-16000 per month and owning more than 2 vehicles is $\frac{1}{96}$.
(v) Total number of families $=2400$
Number of families owning not more than 1 vehicle $=10 + 0 + 1 + 2 + 1 + 160 + 305 + 535 + 469 + 579=2062$
We know that,
Probability of an event=$ \frac{Number \ of \ favourable \ outcomes}{Total \ number \ of \ outcomes}$
Therefore,
Probability that the family is owning not more than 1 vehicle $=\frac{2062}{2400}$
$=\frac{1031}{1200}$
The probability the family is owning not more than 1 vehicle is $\frac{1031}{1200}$.
- Related Articles
- An organisation selected 2400 families at random and surveyed them to determine a relationship between income level and the number of vehicles in a family. The information gathered is listed in the table below:Suppose a family is chosen. Find the probability that the family chosen is(i) earning \( Rs. 10000-13000 \) per month and owning exactly 2 vehicles.(ii) earning \( Rs. 16000 \) or more per month and owning exactly 1 vehicle.(iii) earning less than Rs. 7000 per month and does not own any vehicle.(iv) earning \( Rs. 13000-16000 \) per month and owning more than 2 vehicles.(v) owning not more than 1 vehicle."
- A company selected 2400 families at random and survey them to determine a relationship between income level and the number of vehicles in a home. The information gathered is listed in the table below:If a family is chosen\n
- The fees of a student increases from Rs.1000 per month to Rs. 1200 per month. Find the percentage increase.
- The cost of levelling and turfing a square lawn at Rs. $250$ per $m^2$ is Rs. 13322.50. Find the cost of fencing it at Rs. 5 per metre?
- The following data relates to the expenditure of the families \( A \) and \( B \) per month.
- 1500 families with 2 children were selected randomly and the following data were recorded:If a family is chosen at random\n
- $1500$ families with $2$ children were selected randomly, and the following data were recorded:Number of girls in a family$2$$1$$0$Number of families$475$$814$$211$Compute the probability of a family, chosen at random, having$( i)\ 2$ girls $( ii)\ 1$ girl $( iii)$ No girlAlso check whether the sum of these probabilities is $1$.
- Yasmeen saves Rs 32 during the first month, Rs 36 in the second month and Rs 40 in the third month. If she continues to save in this manner, in how many months will she save Rs 2000?
- In a particular month, a shopkeeper incurs a loss of Rs. 15, by losing 30 paise per pencil. Calculate the number of pencil sold in this month.
- What is the percentage change in the rent of the house if in the month of January it was Rs. 10,000 and in the month of March it is Rs. 15,000?
- Vijay had some bananas, and he divided them into two lots A and B. He sold first lot at the rate of Rs. 2 for 3 bananas and the second lot at the rate of Rs. 1 per banana and got a total of Rs. 400. If he had sold the first lot at the rate of Rs. 1 per banana and the second lot at the rate of Rs. 4 per five bananas, his total collection would have been Rs. 460. Find the total number of bananas he had.
- Using the number line, write the integer which is(i) 4 more than 6(ii) 5 more than \( -6 \)(iii) 6 less than 2(iv) 2 less than \( -3 \)
- Tell what is the profit or loss in the following transactions. Also find profit per cent or loss per cent in each case.(a) Gardening shears bought for Rs. 250 and sold for Rs. 325 .(b) A refrigerater bought for \( Rs. 12,000 \) and sold at \( Rs. 13,500 \).(c) A cupboard bought for \( Rs. 2,500 \) and sold at \( Rs. 3,000 \).(d) A skirt bought for \( Rs. 250 \) and sold at \( Rs. 150 \)
- Hari bought 20 kg of rice at Rs.36 per kg and 25 kg of rice at Rs. 32 per kg. He mixed the two varieties and sold the mixture at Rs. 38 per kg. Find his gain percent in the whole transition.
- The salary for a month of an employee is Rs. 4000. The annual salary of the employee is1) RS 480002) RS 240003) RS 120004) RS 8000
