Prim’s Minimal Spanning Tree

Prim’s minimal spanning tree algorithm is one of the efficient methods to find the minimum spanning tree of a graph. A minimum spanning tree is a sub graph that connects all the vertices present in the main graph with the least possible edges and minimum cost (sum of the weights assigned to each edge).

The algorithm, similar to any shortest path algorithm, begins from a vertex that is set as a root and walks through all the vertices in the graph by determining the least cost adjacent edges.

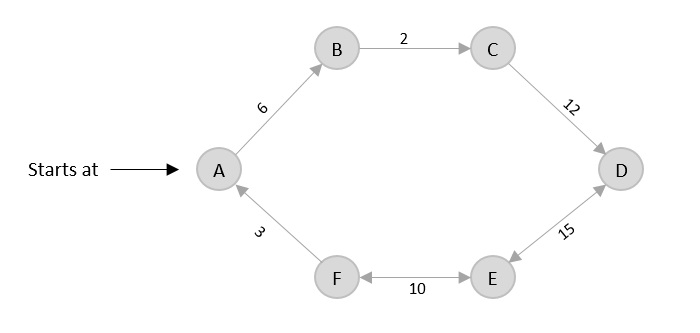
Prim’s Algorithm

To execute the prim’s algorithm, the inputs taken by the algorithm are the graph G {V, E}, where V is the set of vertices and E is the set of edges, and the source vertex S. A minimum spanning tree of graph G is obtained as an output.

Algorithm

• Declare an array visited[] to store the visited vertices and firstly, add the arbitrary root, say S, to the visited array.

• Check whether the adjacent vertices of the last visited vertex are present in the visited[] array or not.

• If the vertices are not in the visited[] array, compare the cost of edges and add the least cost edge to the output spanning tree.

• The adjacent unvisited vertex with the least cost edge is added into the visited[] array and the least cost edge is added to the minimum spanning tree output.

• Steps 2 and 4 are repeated for all the unvisited vertices in the graph to obtain the full minimum spanning tree output for the given graph.

• Calculate the cost of the minimum spanning tree obtained.

Examples

• Find the minimum spanning tree using prim’s method (greedy approach) for the graph given below with S as the arbitrary root.

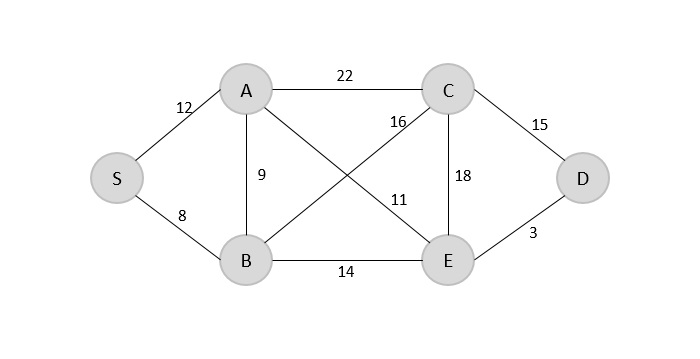
Solution

Step 1

Create a visited array to store all the visited vertices into it.

V = { }

The arbitrary root is mentioned to be S, so among all the edges that are connected to S we need to find the least cost edge.

S → B = 8
V = {S, B}

Step 2

Since B is the last visited, check for the least cost edge that is connected to the vertex B.

B → A = 9
B → C = 16
B → E = 14

Hence, B → A is the edge added to the spanning tree.

V = {S, B, A}

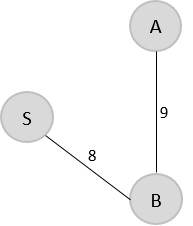
Step 3

Since A is the last visited, check for the least cost edge that is connected to the vertex A.

A → C = 22
A → B = 9
A → E = 11

But A → B is already in the spanning tree, check for the next least cost edge. Hence, A → E is added to the spanning tree.

V = {S, B, A, E}

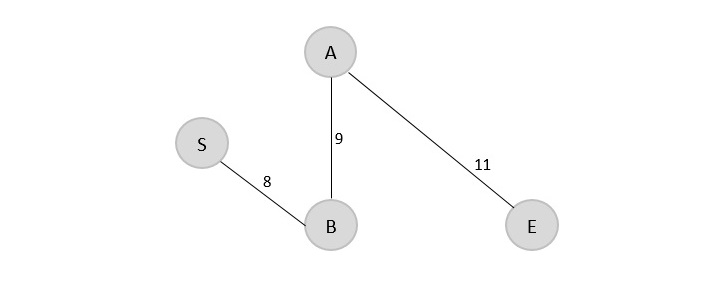
Step 4

Since E is the last visited, check for the least cost edge that is connected to the vertex E.

E → C = 18
E → D = 3

Therefore, E → D is added to the spanning tree.

V = {S, B, A, E, D}

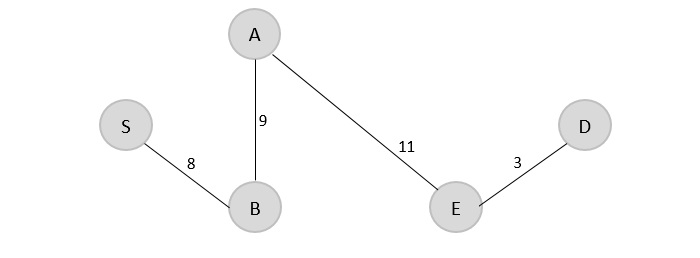
Step 5

Since D is the last visited, check for the least cost edge that is connected to the vertex D.

D → C = 15
E → D = 3

Therefore, D → C is added to the spanning tree.

V = {S, B, A, E, D, C}

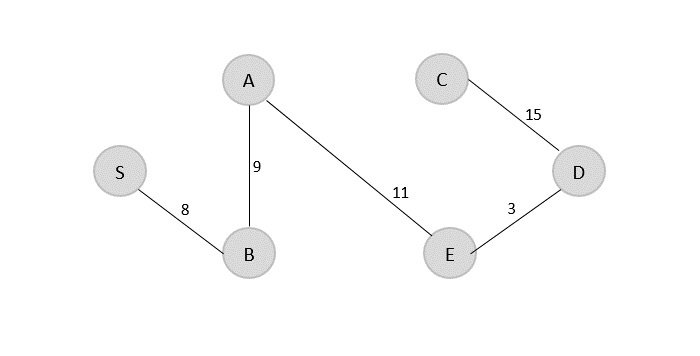
The minimum spanning tree is obtained with the minimum cost = 46

Example

The final program implements Prim’s minimum spanning tree problem that takes the cost adjacency matrix as the input and prints the spanning tree as the output along with the minimum cost.

#include<stdio.h>
#include<stdlib.h>
#define inf 99999
#define MAX 10
int G[MAX][MAX] = {
   {0, 19, 8},
   {21, 0, 13},
   {15, 18, 0}
};
int S[MAX][MAX], n;
int prims();
int main(){
   int i, j, cost;
   n = 3;
   cost=prims();
   printf("Spanning tree:");
   for(i=0; i<n; i++) {
      printf("\n");
      for(j=0; j<n; j++)
         printf("%d\t",S[i][j]);
   }
   printf("\nMinimum cost = %d", cost);
   return 0;
}
int prims(){
   int C[MAX][MAX];
   int u, v, min_dist, dist[MAX], from[MAX];
   int visited[MAX],ne,i,min_cost,j;

   //create cost[][] matrix,spanning[][]
   for(i=0; i<n; i++)
      for(j=0; j<n; j++) {
         if(G[i][j]==0)
            C[i][j]=inf;
         else
            C[i][j]=G[i][j];
         S[i][j]=0;
      }

   //initialise visited[],distance[] and from[]
   dist[0]=0;
   visited[0]=1;
   for(i=1; i<n; i++) {
      dist[i] = C[0][i];
      from[i] = 0;
      visited[i] = 0;
   }
   min_cost = 0; //cost of spanning tree
   ne = n-1; //no. of edges to be added
   while(ne > 0) {

      //find the vertex at minimum distance from the tree
      min_dist = inf;
      for(i=1; i<n; i++)
         if(visited[i] == 0 && dist[i] < min_dist) {
            v = i;
            min_dist = dist[i];
         }
      u = from[v];

      //insert the edge in spanning tree
      S[u][v] = dist[v];
      S[v][u] = dist[v];
      ne--;
      visited[v]=1;

      //updated the distance[] array
      for(i=1; i<n; i++)
         if(visited[i] == 0 && C[i][v] < dist[i]) {
            dist[i] = C[i][v];
            from[i] = v;
         }
      min_cost = min_cost + C[u][v];
   }
   return(min_cost);
}

Spanning tree:
0	0	8	
0	0	13	
8	13	0	
Minimum cost = 26

#include<iostream>
#define inf 999999
#define MAX 10
using namespace std;
int G[MAX][MAX] = {
   {0, 19, 8},
   {21, 0, 13},
   {15, 18, 0}
};
int S[MAX][MAX], n;
int prims();
int main(){
   int i, j, cost;
   n = 3;
   cost=prims();
   cout <<"Spanning tree:";
   for(i=0; i<n; i++) {
      cout << endl;
      for(j=0; j<n; j++)
         cout << S[i][j] << " ";
   }
   cout << "\nMinimum cost = " << cost;
   return 0;
}
int prims(){
   int C[MAX][MAX];
   int u, v, min_dist, dist[MAX], from[MAX];
   int visited[MAX],ne,i,min_cost,j;

   //create cost matrix and spanning tree
   for(i=0; i<n; i++)
      for(j=0; j<n; j++) {
         if(G[i][j]==0)
            C[i][j]=inf;
         else
            C[i][j]=G[i][j];
         S[i][j]=0;
      }

   //initialise visited[],distance[] and from[]
   dist[0]=0;
   visited[0]=1;
   for(i=1; i<n; i++) {
      dist[i] = C[0][i];
      from[i] = 0;
      visited[i] = 0;
   }
   min_cost = 0; //cost of spanning tree
   ne = n-1; //no. of edges to be added
   while(ne > 0) {

      //find the vertex at minimum distance from the tree
      min_dist = inf;
      for(i=1; i<n; i++)
         if(visited[i] == 0 && dist[i] < min_dist) {
            v = i;
            min_dist = dist[i];
         }
      u = from[v];

      //insert the edge in spanning tree
      S[u][v] = dist[v];
      S[v][u] = dist[v];
      ne--;
      visited[v]=1;

      //updated the distance[] array
      for(i=1; i<n; i++)
         if(visited[i] == 0 && C[i][v] < dist[i]) {
            dist[i] = C[i][v];
            from[i] = v;
         }
      min_cost = min_cost + C[u][v];
   }
   return(min_cost);
}

Spanning tree:
0 0 8 
0 0 13 
8 13 0 
Minimum cost = 26

public class prims {
   static int inf = 999999;
   static int MAX = 10;
   static int G[][] = {
       {0, 19, 8},
       {21, 0, 13},
       {15, 18, 0}
   };
   static int S[][] = new int[MAX][MAX];
   static int n;
   public static void main(String args[]) {
      int i, j, cost;
      n = 3;
      cost=prims();
      System.out.print("Spanning tree: ");
      for(i=0; i<n; i++) {
         System.out.println();
         for(j=0; j<n; j++)
            System.out.print(S[i][j] + " ");
      }
      System.out.println("\nMinimum cost = " + cost);
   }
   static int prims() {
      int C[][] = new int[MAX][MAX];
      int u, v = 0, min_dist;
      int dist[] = new int[MAX];
      int from[] = new int[MAX];
      int visited[] = new int[MAX];
      int ne,i,min_cost,j;
      //create cost matrix and spanning tree
      for(i=0; i<n; i++)
         for(j=0; j<n; j++) {
            if(G[i][j]==0)
               C[i][j]=inf;
            else
               C[i][j]=G[i][j];
            S[i][j]=0;
         }
      //initialise visited[],distance[] and from[]
      dist[0]=0;
      visited[0]=1;
      for(i=1; i<n; i++) {
         dist[i] = C[0][i];
         from[i] = 0;
         visited[i] = 0;
      }
      min_cost = 0; //cost of spanning tree
      ne = n-1; //no. of edges to be added
      while(ne > 0) {
         //find the vertex at minimum distance from the tree
         min_dist = inf;
         for(i=1; i<n; i++)
            if(visited[i] == 0 && dist[i] < min_dist) {
               v = i;
               min_dist = dist[i];
            }
         u = from[v];
         //insert the edge in spanning tree
         S[u][v] = dist[v];
         S[v][u] = dist[v];
         ne--;
         visited[v]=1;
         //updated the distance[] array
         for(i=1; i<n; i++)
            if(visited[i] == 0 && C[i][v] < dist[i]) {
               dist[i] = C[i][v];
               from[i] = v;
            }
         min_cost = min_cost + C[u][v];
      }
      return(min_cost);
   }
}

Spanning tree: 
0 0 8 
0 0 13 
8 13 0 
Minimum cost = 26

inf = 999999
MAX = 10
G = [
    [0, 19, 8],
    [21, 0, 13],
    [15, 18, 0]
]
S = [[0 for _ in range(MAX)] for _ in range(MAX)]
n = 3
def main():
    global n
    cost = prims()
    print("Spanning tree: ")
    for i in range(n):
        print()
        for j in range(n):
            print(S[i][j], end=" ")
    print("\nMinimum cost =", cost)
def prims():
    global n
    C = [[0 for _ in range(MAX)] for _ in range(MAX)]
    u, v = 0, 0
    min_dist = 0
    dist = [0 for _ in range(MAX)]
    from_ = [0 for _ in range(MAX)]
    visited = [0 for _ in range(MAX)]
    ne = 0
    min_cost = 0
    i = 0
    j = 0
    for i in range(n):
        for j in range(n):
            if G[i][j] == 0:
                C[i][j] = inf
            else:
                C[i][j] = G[i][j]
            S[i][j] = 0
    dist[0] = 0
    visited[0] = 1
    for i in range(1, n):
        dist[i] = C[0][i]
        from_[i] = 0
        visited[i] = 0
    min_cost = 0
    ne = n - 1
    while ne > 0:
        min_dist = inf
        for i in range(1, n):
            if visited[i] == 0 and dist[i] < min_dist:
                v = i
                min_dist = dist[i]
        u = from_[v]
        S[u][v] = dist[v]
        S[v][u] = dist[v]
        ne -= 1
        visited[v] = 1
        for i in range(n):
            if visited[i] == 0 and C[i][v] < dist[i]:
                dist[i] = C[i][v]
                from_[i] = v
        min_cost += C[u][v]
    return min_cost
#calling  the main() method
main()

Spanning tree: 

0 0 8 
0 0 13 
8 13 0 
Minimum cost = 26